Long-term stability of marine dissolved organic carbon emerges from a neutral network of compounds and microbes
海洋溶解有机碳的长期稳定性源于化合物和微生物的中性网络

Dissolved organic carbon (DOC) represents one of the Earth’s major carbon pools. It contains a similar amount of carbon as the atmosphere and exceeds the amount of carbon bound in marine biomass by more than two-hundred times¹. DOC is mainly produced in the near-surface layers during primary production and grazing processes². Other sources of marine DOC are dissolution from particles², terrestrial and hydrothermal vent input³, and microbial production. Prokaryotes (bacteria and archaea) contribute to the DOC pool via release of capsular material, exopolymers, and hydrolytic enzymes², as well as via mortality (e.g. “viral shunt”). Prokaryotes are also the main decomposers of DOC, although for some of the most recalcitrant forms of DOC very slow abiotic degradation in hydrothermal systems² or possibly sorption to sinking particles⁴ may be the main removal mechanism. Mechanistic knowledge about DOC-microbe-interactions is crucial to understand the cycling and distribution of this active carbon reservoir.
溶解有机碳（DOC）是地球上主要的碳库之一。它含有与大气中相似数量的碳，并且超过海洋生物量中固定碳的数量两百多倍。DOC主要在近表层产生，通过初级生产和捕食过程产生。海洋DOC的其他来源包括来自颗粒物的溶解、陆地和热液喷口输入以及微生物产生。原核生物（细菌和古菌）通过释放胶囊物质、外聚物和水解酶，以及通过死亡（例如“病毒转运”）来贡献DOC库。原核生物也是DOC的主要分解者，尽管对于一些最难降解的DOC形式，在热液系统中可能存在非常缓慢的非生物降解，或者可能是吸附到下沉颗粒物的主要去除机制。了解DOC与微生物相互作用的机制性知识对于理解这个活跃碳库的循环和分布至关重要。

DOC is conceptually divided into labile DOC, which is rapidly taken up by heterotrophic microbes, and the recalcitrant DOC reservoir, which has accumulated in the ocean (following the definition by Hansell⁴). As a consequence of its recalcitrance, the accumulated DOC reaches average radiocarbon ages of ~1,000–4,000 years in surface waters and ~3,000–6,000 years in the deep ocean⁵, indicating that it persists through several deep ocean mixing cycles of ~300–1,400 years each⁶. Behind these average radiocarbon ages, a large spectrum of ages is hidden: Follett et al.⁷ showed that DOC comprises a fraction of modern radiocarbon age, as well as DOC reaching radiocarbon ages of up to 12,000 years.
DOC在概念上分为不稳定的DOC和难降解的DOC储量。不稳定的DOC被异养微生物迅速吸收，而难降解的DOC储量则积累在海洋中（根据Hansell的定义）。由于其难降解性，积累的DOC在表层水体中的平均放射性碳年龄约为1,000-4,000年，在深海中约为3,000-6,000年，这表明它在每个约300-1,400年的深海混合周期中持续存在。在这些平均放射性碳年龄之后，隐藏着一个广泛的年龄范围：Follett等人表明，DOC包含一部分现代放射性碳年龄的物质，以及放射性碳年龄高达12,000年的DOC。

The surprising resistance of high concentrations of DOC to microbial degradation has been addressed by several hypotheses (e.g. Dittmar⁸). The prevalent notion is that the recalcitrant fraction of DOC has certain chemical properties, which prevent decomposition by microbes (“intrinsic stability hypothesis”). An alternative or additional explanation is given by the “dilution hypothesis”, that all compounds are labile, but exist in concentrations individually too low to sustain microbial populations but collectively form a large pool⁹. The dilution hypothesis found support in recent experimental and theoretical studies^10,11, which sparked a vivid discussion of its plausibility^{5,12,13,14,15,16}. To date, little is known about the substrate affinity of microbes towards DOC compounds¹⁷, therefore both the intrinsic stability of compounds and dilution limitation are possible scenarios.
高浓度的溶解性有机碳（DOC）对微生物降解的惊人抵抗性已经被几个假设所解释（例如Dittmar）。普遍的观点是，DOC的难降解部分具有某些化学性质，这些性质阻止了微生物的分解（“内在稳定性假设”）。另一个或附加的解释是“稀释假设”，即所有化合物都是易降解的，但个别浓度太低以至于无法维持微生物群落，但总体上形成了一个大的池。稀释假设在最近的实验和理论研究中得到了支持，引发了对其可信度的热烈讨论。迄今为止，对微生物对DOC化合物的底物亲和性知之甚少，因此化合物的内在稳定性和稀释限制都是可能的情景。

Various models have been used to approach the unanswered questions regarding the stability and decomposition of dissolved organic matter (DOM), i.e. of dissolved organic carbon, nitrogen, and phosphorus. In existing DOM models, DOM stability is assumed to result from either intrinsic recalcitrance (RDOC_t¹⁸) or dilution limitation (RDOC_c¹⁸). Intrinsic recalcitrance is reflected in DOM models in the form of a DOM fraction unavailable to microbial degradation^19,20,21,22, a fraction of DOC which is assumed to be less degradable^23,24,25, or in distinct DOM pools with fixed life-times and no exchange between them^1,26. Dilution limitation is incorporated into DOM models in the form of a lower concentration limit, below which microbial uptake of DOM is suppressed^14,22.
关于溶解有机物（DOM）的稳定性和分解的未解之谜，已经使用了各种模型进行研究，包括溶解有机碳、氮和磷。在现有的DOM模型中，DOM的稳定性被认为是由内在难降解性（RDOC _t ¹⁸ ）或稀释限制（RDOC _c ¹⁸ ）所导致的。内在难降解性在DOM模型中以一种对微生物降解不可利用的DOM分数的形式体现 ^19,20,21,22 ，这是一种被认为不易降解的DOC分数 ^23,24,25 ，或者以具有固定寿命且彼此之间没有交换的不同DOM池的形式体现 ^1,26 。稀释限制以DOM模型中的浓度下限的形式体现，低于该下限时，微生物对DOM的摄取被抑制 ^14,22 。

The objective of our study was to numerically evaluate long-term (years to millennia) DOC stability and dynamics in a theoretical scenario of complete neutrality of compounds. We model DOC dynamics by neglecting any structural reactivity differences among compounds. We test whether this extreme scenario can lead to a realistic size and age of the DOC pool, despite the lack of any abiotic stabilization mechanism of DOC. In contrast to existing models, we model the dynamics of DOC and microbes without assuming intrinsic recalcitrance, and without a mathematical formulation of a concentration limit for microbial DOC uptake. The uptake of individual DOC constituents is slowed down at low concentrations, according to the well-established Michaelis-Menten kinetic (e.g. Polimene et al.²³, Grégoire et al.²⁷), but it is not suppressed completely, as long as the microbial population persists. In this regard, the model is different from the “dilution hypothesis”¹⁰, which proposes a fixed lower limit of bulk DOC below which no DOC uptake can take place. Our experiments are not designed or intended to prove or disprove the existence of structurally-recalcitrant molecules, which exist in the ocean for example in the form of black carbon^28,29. The aim of this study is to explore the possible implications of a large diversity of equally reactive compounds on the size and age of the marine DOC pool.
我们研究的目标是在理论上完全中性化的化合物情景中，对长期（数年至千年）的DOC稳定性和动态进行数值评估。我们在模型中忽略了化合物之间的结构反应性差异，来模拟DOC的动态。我们测试这种极端情况是否能够在缺乏任何非生物稳定机制的情况下，导致DOC池的实际大小和年龄。与现有模型不同的是，我们在模型中对DOC和微生物的动态进行建模时，不假设固有的难降解性，并且没有数学公式来限制微生物对DOC的摄取浓度。根据已经确立的Michaelis-Menten动力学（例如Polimene等人，Grégoire等人），低浓度下单个DOC成分的摄取速度会减慢，但只要微生物群体存在，摄取并不会完全停止。在这方面，该模型与“稀释假设”不同，后者提出了一个固定的DOC下限，低于该下限时不会发生DOC摄取。我们的实验并不旨在证明或否定存在于海洋中的结构难降解分子的存在，例如黑碳的形式。本研究的目的是探讨等反应性化合物的大多样性对海洋DOC池的大小和年龄可能产生的影响。

We emphasize that simplification is one of the crucial steps in model formulation, allowing to identify causal relationships within complex systems by purposefully excluding most of the natural variety and focusing on selected key processes. Therefore, our model neglects abiotic production and removal processes, direct interaction among microbes, and higher trophic levels, for example. By representing intricate geochemical and ecological interactions in a simplified but mechanistic manner, our model helps to identify fundamental and emergent properties the DOC reservoir that serve to guide further observations and experiments.
我们强调简化是模型制定中的关键步骤之一，通过有意地排除大部分自然变异并专注于选择的关键过程，可以识别复杂系统中的因果关系。因此，我们的模型忽略了非生物产生和去除过程、微生物之间的直接相互作用以及更高的营养级别等。通过以简化但机械化的方式表示复杂的地球化学和生态学相互作用，我们的模型有助于识别影响进一步观察和实验的DOC储量的基本和新兴特性。

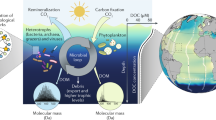
We model the degradation and accumulation of marine DOC, assuming a large network of DOC compounds and microbes (Fig. 1). The microbes take up DOC, fix a fraction of that carbon into their biomass, respire a fraction of it to inorganic carbon (CO₂), and release the remaining carbon as transformed DOC compounds back to the DOC pool. Microbes also contribute to the formation of DOC via lysis. New DOC is supplied from unspecified sources external to the microbe-resource network, which may include primary production, particle dissolution, or hydrothermal vents.
我们对海洋DOC的降解和累积进行建模，假设存在大量的DOC化合物和微生物网络（图1）。微生物吸收DOC，将其中一部分碳固定到它们的生物量中，将其中一部分呼吸成无机碳（CO ₂ ），并将剩余的碳释放为转化后的DOC化合物返回到DOC池中。微生物还通过溶解对DOC的形成做出贡献。新的DOC来自于未指明的微生物资源网络外部来源，可能包括初级生产、颗粒溶解或热液喷口。

Scheme of the DOC-microbe-interaction model. The model predicts the carbon concentration of individual microbial units B_i, individual DOC compound units D_j, and the inorganic carbon pool I (note that due to the study focus the results for I are not shown). The arrows depict fluxes of carbon, where the width of the arrow indicates the strength of the flux. The carbon consumed is split among three pathways: respiration, microbial growth, and release of transformed compounds. The transformation of compounds is defined by the uptake and release matrices (dotted circles), which transfer carbon between pools. Each microbial unit takes up and releases a specific set of compounds. The supply of DOC represents an external source of DOC, e.g. from primary production.
DOC-微生物相互作用模型的方案。该模型预测了个体微生物单位B的碳浓度，个体DOC化合物单位D的碳浓度，以及无机碳池I（注意，由于研究重点，I的结果未显示）。箭头表示碳的流动，箭头的宽度表示流动的强度。消耗的碳分为三个途径：呼吸作用、微生物生长和转化化合物的释放。化合物的转化由摄取和释放矩阵（虚线圆圈）定义，这些矩阵在池之间转移碳。每个微生物单位摄取和释放一组特定的化合物。DOC的供应代表了外部的DOC来源，例如来自初级生产。

Full size image

The model comprises a variety of “microbial units”, which each take up and release a specific set out of the modelled “DOC compound units”. A microbial unit is here defined as the group of heterotrophic bacteria or archaea that can take up the same groups of compounds. The DOC compounds are grouped hypothetically by structural properties, which govern uptake. Each microbial unit takes up and releases a specific set of compound units, forming a complex, bipartite network of DOC-microbe-interactions (see Material and Methods for an illustrative example). Assuming neutral reactivity, we neglect any reactivity differences among the DOC compounds. Accordingly, the uptake rate of a compound depends exclusively on its concentration (Michaelis-Menten kinetic). At low concentrations, the uptake is slower, but there is no physiological lower limit for microbial DOC uptake. Published estimates served as a basis for the parameterization of e.g. the uptake and mortality rate of microbes (for details see Materials and Methods). Unfortunately, the exact values of the model parameters are poorly constrained for marine organisms, especially for the deep sea and for archaea. To address this uncertainty, we tested the sensitivity of model results using a range of model parameters.
该模型包括各种“微生物单元”，每个单元都吸收和释放模拟的“DOC化合物单元”中的特定集合。微生物单元在这里被定义为能够吸收相同化合物组的异养细菌或古菌群。假设DOC化合物根据其结构特性被假设分组，这些特性将影响吸收。每个微生物单元吸收和释放特定的化合物单元集合，形成了复杂的DOC-微生物相互作用的双部分网络（请参阅材料和方法提供的示例）。假设中性反应，我们忽略了DOC化合物之间的反应差异。因此，化合物的吸收速率完全取决于其浓度（米氏-蒙特宁动力学）。在浓度较低时，吸收速度较慢，但微生物对DOC的摄取没有生理学的下限。已发表的估计值为参数化提供了基础，例如微生物的摄取和死亡速率（有关详细信息，请参阅材料和方法）。不幸的是，就海洋生物而言，尤其是对于深海和古菌来说，模型参数的确切值受到很大的限制。为了解决这一不确定性，我们使用一系列模型参数来测试模型结果的敏感性。

We aim to reproduce essential features of DOC in the ocean with our conceptual model. The model should thus be in agreement with the following observations concerning DOC: (i) microheterotrophs form recalcitrant DOC^30,31,32,33, which is (ii) taken up if concentrated¹⁰, (iii) DOC concentrations range between 30–80 mmolC/m³ in the ocean^1,34, and (iv) DOC shows a broad spectrum of radiocarbon ages in the deep sea from 10 to ~10,000 years⁷. Targets of our numerical model are not short-term dynamics of DOC turnover, e.g. during and after a phytoplankton bloom. On these time scales, the affinity of microbial taxa to specific substrate compounds is clearly important. For the purpose of our study we purposefully refrain from ascribing different affinities to different DOC compounds, in order to test the explanatory power of a model with neutral reactivity.
我们的概念模型旨在复制海洋中DOC的基本特征。因此，该模型应符合以下关于DOC的观测结果：(i) 微异养物种形成难降解的DOC， (ii) 浓度高时被摄取， (iii) 海洋中DOC浓度在30至80 mmolC/m之间， (iv)深海中DOC的放射性碳年龄范围广泛，从10年到约10,000年。我们数值模型的目标不是DOC周转的短期动态，例如，光合浮游植物暴发期间和之后的动态。在这些时间尺度上，微生物分类对特定底物化合物的亲和力显然很重要。为了测试具有中性反应性的模型的解释能力，我们在研究中故意不将不同的DOC化合物归因于不同的亲和力。

Formation of recalcitrant DOC in incubation experiments
在孵育实验中形成难降解DOC的过程

The amount of (apparently) recalcitrant DOC is determined after 100 simulation years of degradation of an initial DOC pulse (Fig. 2). This is done for three different scenarios: (a) no supply of DOC compounds from primary production, representing a bottle experiment, (b) low supply of DOC, representing low nutrient regions, and (c) high supply of DOC, representing bloom conditions.
在初始DOC脉冲降解100个模拟年后，确定（表面上）难降解DOC的数量（图2）。这是针对三种不同情景进行的：（a）没有来自初级生产的DOC化合物供应，代表瓶子实验，（b）低DOC供应，代表低营养区域，和（c）高DOC供应，代表盛开条件。

Formation of recalcitrant DOC in incubation experiments. (A) Total DOC concentration and (C) total microbial biomass is shown over simulation time, for three levels of external DOC supply: high (bold lines), low (thin lines), and no supply (dashed lines). Note that in panels (A,C) the horizontal axis is broken after five years, continuing to show years 95–100. (B,D) Full time-series is shown on a double-logarithmic scale.
在孵育实验中形成难降解DOC的过程。 （A）显示了模拟时间内的总DOC浓度和（C）总微生物生物量，对应三个不同水平的外部DOC供应：高（粗线），低（细线）和无供应（虚线）。请注意，在面板（A，C）中，水平轴在五年后中断，继续显示95-100年。（B，D）在双对数刻度上显示完整的时间序列。

Full size image

DOC and microbial biomass concentrations are in the range of observed values for each of the three scenarios (Fig. 2). Without any DOC supply, the percentage of recalcitrant DOC (~16% of initially provided DOC, corresponding to 13 mmolC/m³) is comparable to experimental results (5–10% for simple substrates^30,31,32; 35–80% for more diverse substrates^32,33). The model is designed to study the long-term stability of DOC in the ocean, and it is parameterized according to published values for deep-sea microbial communities. As such, it overestimates the time scale of initial DOC consumption (~200 simulated days, reported degradation time-scales are ~3–21 days^30,31,32,33). Due to the limited amount of carbon available in the scenario of no supply, the microbial community continuously declines in biomass and becomes vanishingly small after about one year.
DOC和微生物生物量浓度在每个场景中的观察值范围内（图2）。在没有任何DOC供应的情况下，难降解DOC的百分比（初始提供的DOC的约16%，相当于13 mmolC/m ³ ）与实验结果相当（简单底物为5-10%，更多样化底物为35-80% ^{30,31,32 32,33} ）。该模型旨在研究海洋中DOC的长期稳定性，并根据已发表的深海微生物群落的数值进行参数化。因此，它高估了初始DOC消耗的时间尺度（约200个模拟天，报告的降解时间尺度为3-21天 ^30,31,32,33 ）。由于在没有供应的情况下碳的数量有限，微生物群落的生物量不断下降，并在大约一年后变得微不足道。

For low and high DOC supply rates, the DOC concentration after 100 simulation years is close to values observed in the deep Pacific Ocean (~43% of initial DOC, corresponding to 35 mmolC/m^3 1). The final microbial biomass is close to values observed for free-living bacteria in the bathypelagic ocean (0.12 mmolC/m^3 35), for the low-supply-scenario and in the range of values observed for the epipelagic ocean (1.21 mmolC/m^3 35), for the high-supply-scenario (see Supplement Fig. S1 for the concentration distribution of compounds at the end of the simulation).
对于低和高DOC供应速率，100年模拟后，DOC浓度接近于在太平洋深层观察到的值（初始DOC的大约43%，相当于35 mmolC/m ^{3 1} ）。最终微生物生物量接近于在深层海洋中观察到的游离细菌的值（0.12 mmolC/m ^{3 35} ）对于低供应情景，以及在表层海洋中观察到的值范围内（1.21 mmolC/m ^{3 35} ）对于高供应情景（请参见补充图S1了解模拟结束时化合物的浓度分布）。

Our model shows the formation of an (apparently) recalcitrant DOC pool from bioavailable DOC. At the end of the virtual incubations, a significant fraction of the initial DOC concentration is left, independent of the supply rate (Fig. 2, high or low DOC supply). The microbial production of a heterogeneous mixture of recalcitrant DOC compounds has been observed in laboratory experiments^30,31.
我们的模型显示，可生物利用的DOC形成了一个（表面上）难以降解的DOC池。在虚拟孵育结束时，无论供应速率如何（图2，高或低DOC供应），初始DOC浓度的相当一部分仍然存在。实验室实验证明，微生物产生了一种多样的难以降解的DOC化合物混合物。

Recalcitrance of DOC in the model results from two different mechanisms: (a) if there is no supply of DOC, the microbes go almost extinct after the initial bloom and thus “leave behind” a residual concentration of DOC, and (b) if there is supply of DOC, microbes maintain a stable population on the long-term and therefore the DOC concentration reaches a steady-state where uptake, release, and supply are balanced (see double-logarithmic inlays in Fig. 2).
模型中DOC的难降解性来自两种不同的机制：(a)如果没有DOC的供应，微生物在初始盛开后几乎灭绝，因此“留下”了残留的DOC浓度；(b)如果有DOC的供应，微生物在长期内维持稳定的种群，因此DOC浓度达到一个稳定状态，其中摄取、释放和供应保持平衡（见图2中的双对数插图）。

On a time scale of years to decades, the amount of recalcitrant DOC depends on the supply rate (Fig. 2). The reported accumulation of DOC in the surface layer of the Atlantic Ocean depends on the rate of New Primary Production³⁶. Our model is in agreement with this observation: on short time scales (<50 years, i.e. shorter than the residence time of surface water), DOC concentrations increase with its supply rate. On longer time-scales, however, the amount of (apparently) recalcitrant DOC does not depend on the supply rate (Fig. 2A,C). In the future centuries, increased levels of CO₂ in the atmosphere might enhance the algal production of DOM^37,38, and thus the supply rate of DOC. Experiments showed that there is no significant change in DOC concentration under increased CO₂ levels³⁹. Our model supports this observation: increased primary production, represented by elevated supply rates, does not affect long-term DOC concentrations. However, it increases the microbial biomass by orders of magnitude (Fig. 2B,C, and Supplement Fig. S2J). The “additional” DOC from increased primary production is thus partially stored in the biomass of consumers.
在年到十年的时间尺度上，难降解的溶解有机碳（DOC）的数量取决于供应速率（图2）。报道的大西洋表层海洋中DOC的积累取决于新初级生产的速率 ³⁶ 。我们的模型与这一观察结果一致：在短时间尺度（<50年，即短于表层水停留时间），DOC浓度随其供应速率增加。然而，在较长时间尺度上，（明显）难降解的DOC的数量不取决于供应速率（图2A、C）。在未来几个世纪中，大气中增加的CO ₂ 水平可能会增强DOM ^37,38 的藻类生产，从而增加DOC的供应速率。实验证明，在增加的CO ₂ 水平下，DOC浓度没有显著变化 ³⁹ 。我们的模型支持这一观察结果：增加的初级生产，即提高的供应速率，不会影响长期的DOC浓度。然而，它会使微生物生物量增加数个数量级（图2B、C和补充图S2J）。因此，增加的初级生产所带来的“额外”DOC部分储存在消费者的生物量中。

Size of the DOC reservoir: A sensitivity analysis
DOC储量的大小：敏感性分析

To assess the sensitivity of the DOC reservoir to model parameters, we determine the steady-state DOC concentration for a range of parameter values (Fig. 3, see supplement for a sensitivity analysis of microbial biomass, mean DOC age, and other model parameters, Fig. S2, Fig. S3, Fig. S4). While one parameter is varied, the others are kept at their default value. Overall, the size of the DOC reservoir changes by a factor of about 0.5–1.5 across all tested parameter ranges. The model results are thus robust to moderate variation of the parameters. Large deviations only occur when varying parameters by orders of magnitude. The model predicts steady-state DOC concentrations between 17–74 mmolC/m³, covering the range of marine DOC observations^1,34.
为了评估DOC储量对模型参数的敏感性，我们确定了一系列参数值下的稳态DOC浓度（图3，请参阅补充材料以获取对微生物生物量、平均DOC年龄和其他模型参数的敏感性分析，图S2，图S3，图S4）。在变化一个参数的同时，其他参数保持默认值。总体而言，在所有测试的参数范围内，DOC储量的大小变化约为0.5-1.5倍。因此，模型结果对参数的适度变化是稳健的。只有当参数变化数个数量级时，才会出现较大的偏差。模型预测稳态DOC浓度在17-74 mmolC/m ³ 之间，涵盖了海洋DOC观测范围 ^1,34 。

Size of the DOC reservoir. The total concentration of DOC after 20,000 simulation years for varying parameter values: (A) the microbial uptake rate ρ, (B) the microbial mortality rate µ, (C) the number of DOC units n, and (D) the total supply rate of DOC s (see Supplement, Fig. S4 for the remaining parameters). Each simulation was repeated 50 times, the green dots indicate the mean, the light green area represents the minimum and maximum concentrations from the 50 runs. The black triangle indicates the default value of the parameter. The hatched region indicates the range of DOC concentrations typically observed in the surface ocean, the cross-hatched region indicates typical deep sea DOC values^1,34. For more scenarios we refer to the Supplementary Material.
DOC储量的大小。在不同参数值下，经过20,000个模拟年后的总DOC浓度：（A）微生物摄取速率ρ，（B）微生物死亡率µ，（C）DOC单位数n，以及（D）DOC的总供应速率s（有关其余参数，请参见补充材料，图S4）。每个模拟重复了50次，绿色点表示平均值，浅绿色区域表示50次运行中的最小和最大浓度。黑色三角形表示参数的默认值。阴影区域表示表面海洋中通常观察到的DOC浓度范围，斜纹区域表示典型的深海DOC值。更多情景请参阅补充材料。

Full size image

Increasing parameters that fuel microbial growth (i.e. maximum uptake rate ρ, the proportion of substrates taken up per microbe n_U/n, and the microbial growth efficiency) decreases the size of the DOC reservoir (Fig. 3A, and Supplement Fig. S4). In contrast, increasing parameters that constrain microbial biomass (i.e. mortality rate µ and half-saturation constant κ) increases the size of the DOC reservoir (Fig. 3B, and Supplement Fig. S4).
增加促进微生物生长的参数（即最大摄取速率ρ、每个微生物摄取底物的比例n _U /n和微生物生长效率）会减小DOC储量的大小（图3A和补充图S4）。相反，增加限制微生物生物量的参数（即死亡率µ和半饱和常数κ）会增加DOC储量的大小（图3B和补充图S4）。

The size of the DOC reservoir also increases with the diversity of DOC compounds (number of DOC compound units n, Fig. 3C). The microbial community reduces the concentration of a DOC compound until the energetic costs for survival and maintenance (i.e. respiration, mortality) exceed the amount of carbon gained through uptake of this compound unit (according to resource competition theory⁴⁰). Each compound is therefore left over at its limiting concentration (i.e. the equilibrium DOC level D*). The higher the DOC diversity, the more compounds at their individual limiting concentrations add up to a larger total DOC pool.
DOC储量的大小也随着DOC化合物的多样性增加而增加（DOC化合物单位数n的数量，图3C）。微生物群落降低DOC化合物的浓度，直到生存和维持的能量成本（即呼吸、死亡）超过通过摄取该化合物单位获得的碳量（根据资源竞争理论）。因此，每种化合物都以其限制浓度（即平衡DOC水平D*）剩余。DOC多样性越高，越多的化合物以其各自的限制浓度相加形成更大的总DOC池。

It might seem surprising that the supply rate of DOC does not affect the steady-state DOC concentration (Fig. 3D). However, because DOC is supplied at a constant rate, microbes adapt to this level of carbon available and are able to maintain higher levels of biomass (see Supplement Fig. S2F). Hence, the supplied carbon is converted to microbial biomass, leaving the steady-state DOC concentration unaltered. Note that while this is true on long time-scales (millennia, or steady-state, respectively), on short time-scales (years to decades) the size of the DOC reservoir depends on the amount of supply from primary production (Fig. 2A).
供应速率影响不了稳态DOC浓度可能看起来令人惊讶（图3D）。然而，由于DOC以恒定速率供应，微生物适应了这个水平的碳可利用性，并能维持较高水平的生物量（见补充图S2F）。因此，提供的碳被转化为微生物生物量，使得稳态DOC浓度保持不变。请注意，虽然这在长时间尺度上是正确的（千年或稳态，分别），但在短时间尺度上（几年至几十年）DOC储集库的大小取决于来自初级生产的供应量（图2A）。

We identified the number of compound units n, the number of compounds taken up per microbial unit n_U, and the half-saturation constant κ as important factors for the steady-state DOC concentration, while being the least constrained by published referenced values. An increase in the number of compound units n can be “compensated” (i.e. the steady-state DOC concentration can be preserved) by either an increase in the number of taken-up compound units per microbial unit n_U by the same factor, or a proportional decrease in the half-saturation constant κ (see Supplement Fig. S5C).
我们确定了复合单位数n、每个微生物单位吸收的复合物数n _U ，以及半饱和常数κ作为稳态DOC浓度的重要因素，而这些因素受到已发表的参考值的限制最少。复合单位数n的增加可以通过以下两种方式“补偿”（即可以保持稳态DOC浓度）：要么通过相同因子增加每个微生物单位吸收的复合物数n _U ，要么通过按比例减少半饱和常数κ（见补充图S5C）。

For a general overview of the sensitivity of the DOC concentration to parameter variations at steady-state we take advantage of the mean field theory. According to this theory, our interactive network model collapses into a simple equation if total neutrality is assumed (see supplement for details). In that case, the size of the DOC reservoir in steady-state D* can be estimated as
对于稳态下DOC浓度对参数变化的敏感性的一般概述，我们利用平均场理论。根据这个理论，如果假设总中性（详见补充说明），我们的交互网络模型会简化为一个简单的方程。在这种情况下，稳态下DOC储量的大小D*可以估计为

This equation summarizes the influence of model parameters on the long-term DOC concentration D*. It is proportional to mortality rate µ, the half-saturation constant of DOC uptake κ, and the diversity of DOC compounds n. The long-term DOC concentration is constrained by the microbial growth efficiency η, the maximum uptake rate ρ, and the number of compound units consumed per microbial unit n_U.
这个方程总结了模型参数对长期DOC浓度D*的影响。它与死亡率µ、DOC摄取的半饱和常数κ和DOC化合物的多样性n成正比。长期DOC浓度受微生物生长效率η、最大摄取速率ρ和每个微生物单位消耗的化合物单位数n _U 的限制。

Note that the equilibrium DOC level D* is not a parameter of the model, but a consequence of the basic ecological processes that were modelled. This simple equation describes the long-term DOC concentration based on the turnover of an average DOC compound by an average microbial unit. For other, more detailed model outcomes, e.g. the distribution of concentration across compounds and microbes, or the age distribution of DOC, the full network model is required. The equilibrium concentration D*, which can be derived without simulation, is a minimum DOC estimate. In the case of non-neutral DOC uptake (reactivity classes in alternative model set-up, see supplement), the final DOC concentration is equal to or higher than D*. Substrate diversity in relation to microbial uptake capabilities (n/n_U) emerges as one of the key drivers that determine the size of the DOC pool in the ocean. At a fixed microbial uptake capability (fixed number of DOC compound units taken up per bacterial unit n_U), an increase in DOC compound diversity implies an increase in the long-term DOC concentration D*.
请注意，平衡的DOC水平D*不是模型的参数，而是对建模的基本生态过程的结果。这个简单的方程描述了基于平均微生物单位对平均DOC化合物的周转的长期DOC浓度。对于其他更详细的模型结果，例如化合物和微生物浓度的分布，或者DOC的年龄分布，需要使用完整的网络模型。可以不经过模拟推导出的平衡浓度D*是最小的DOC估计。在非中性DOC摄取的情况下（在替代模型设置中的反应性类别，请参见补充说明），最终的DOC浓度等于或高于D*。与微生物摄取能力相关的底物多样性（n/n _U ）成为决定海洋DOC池大小的关键驱动因素之一。在固定的微生物摄取能力（每个细菌单位摄取的DOC化合物单位数n _U ）下，DOC化合物多样性的增加意味着长期DOC浓度D*的增加。

Millennial scale stability of DOC
千禧年尺度的DOC稳定性

To approximate the persistence of DOC in the ocean, the radiocarbon age of DOC compounds is simulated in an isolated, Lagrangian water parcel moving along the deep oceanic circulation (Fig. 4). The total supply rate of DOC is variable over time, to mimic the changes between the rapid DOC supply in surface waters and the much slower DOC supply in the deep-sea. To reflect the selective production of DOC compounds by an algal bloom, only a subset of compounds is supplied (3 out of 100, value chosen to represent a scenario where most compounds are exclusively produced by microbial consumers).
为了近似海洋中DOC的持久性，模拟了一个孤立的拉格朗日水团沿着深海环流移动时DOC化合物的放射性碳年龄（图4）。DOC的总供应速率随时间变化，以模拟表层水体中快速DOC供应和深海中较慢的DOC供应之间的变化。为了反映藻华对DOC化合物的选择性产生，只提供了一部分化合物（100个中的3个，该值被选择为代表大多数化合物仅由微生物消费者产生的情况）。

Illustration of millennial scale DOC stability simulation. The aging of DOC is simulated in a water parcel moving along the ocean circulation. The journey of the water parcel in 25 overturning cycles is simulated by applying a varying DOC supply: at the beginning of each cycle, one year of surface conditions is simulated in the form of high supply of DOC, whereas for the following 899 years low supply rates of DOC simulate deep-sea conditions. The variations in total supply rate affect the total DOC concentration and the concentration-weighted mean DOC age, for results see Fig. 5.
千禧年尺度DOC稳定性模拟的插图。在海洋环流中移动的水团中模拟DOC的老化过程。通过应用不同的DOC供应来模拟水团在25个翻转周期中的旅程：在每个周期的开始，模拟一年的表面条件，以高供应的DOC形式，而在接下来的899年中，以低供应速率的DOC模拟深海条件。总供应速率的变化影响总DOC浓度和浓度加权平均DOC年龄，结果见图5。

Full size image

The concentration of DOC and microbial biomass in the overturning cycles is close to observations. During the surface-supply-regime, the total DOC concentration corresponds to values observed in most of the surface and mesopelagic ocean^1,34 (Fig. 5A,B; see Supplement Fig. S6 for biomass). During the transition from the surface to the deep-sea supply regime, the DOC concentration slowly decreases to values representative for North Pacific deep waters¹. The dynamics of DOC are slightly different among the overturning cycles, indicating that the duration of one overturning cycle did not allow for complete equilibration of the system within one cycle.
DOC和微生物生物量在翻转周期中的浓度接近观测值。在表层供应制度期间，总DOC浓度与大部分表层和中层海洋中观测到的值相对应 ^1,34 （图5A，B；有关生物量，请参见补充图S6）。在从表层向深海供应制度的过渡中，DOC浓度缓慢下降，达到代表北太平洋深水的值 ¹ 。DOC的动态在翻转周期中略有不同，表明一个翻转周期的持续时间不足以使系统在一个周期内完全平衡。

Millennial scale stability of DOC. The variations in total supply rate along the simulated Lagrangian ocean circulation (see Fig. 4) influence (A, B) the total DOC concentration and (D,E) the concentration-weighted mean DOC age. The bold, black line indicates the concentration-weighted mean of the average compound ages. The thin coloured lines show the mean compound age of the individual DOC compound units. Their colour indicates the proportion of microbially-reworked to primary-produced compounds: red compounds are directly supplied via primary production; blue compounds are exclusively microbially reworked. The left column of panels shows the first 20 overturning cycles, during which the system approaches a dynamic equilibrium. The right column shows the last five overturning cycles of the equilibrated system in detail. The distribution of DOC ages across compounds in the last overturning cycle is shown for the (C) surface and deep (F) supply regime. The dotted lines indicate the concentration-weighted mean age.
千年尺度的DOC稳定性。模拟的拉格朗日洋流（见图4）中的总供应速率的变化影响（A，B）总DOC浓度和（D，E）浓度加权平均DOC年龄。粗黑线表示平均化合物年龄的浓度加权平均值。细色线显示各个DOC化合物单位的平均化合物年龄。它们的颜色表示经微生物重组与原生产化合物的比例：红色化合物是通过原生产直接供应的；蓝色化合物完全是经微生物重组的。左列面板显示了前20个翻转周期，在此期间系统接近动态平衡。右列显示了平衡系统的最后五个翻转周期的详细情况。最后一个翻转周期中化合物的DOC年龄分布显示在（C）表层和深层（F）供应制度中。虚线表示浓度加权平均年龄。

Full size image

The mean DOC age increases over time, until it equilibrates after about 20 cycles at an age of ~8,200 years (Fig. 5D,E). The DOC age temporarily decreases at the start of each new overturning cycle, as the water parcel receives high rates of new DOC with zero ‘age’. The difference in the age of surface DOC (~5,800 years, Fig. 5C) and deep sea DOC (~8,000 years, Fig. 5F) is similar to the age difference observed in the ocean (~1,000–3,000 years⁵). The age of DOC compounds in the model ranges from less than hundred to several thousand years (~80–11,000 years). The age spectrum of modelled DOC compounds is thus in agreement with observations of marine DOC, which shows a broad spectrum of radiocarbon ages from modern up to 12,000 years^3,7.
平均DOC年龄随时间增长，直到在大约20个循环后达到平衡，年龄约为8,200年（图5D，E）。每次新的翻转循环开始时，DOC年龄暂时下降，因为水团接收到高速率的新DOC，其年龄为零。表层DOC（约5,800年，图5C）和深海DOC（约8,000年，图5F）的年龄差异类似于海洋中观察到的年龄差异（约1,000-3,000年）。模型中DOC化合物的年龄范围从几十年到几千年不等（约80-11,000年）。模拟的DOC化合物年龄谱与海洋DOC的观测结果一致，显示出从现代到12,000年的广泛放射性碳年龄谱。

Two groups of compounds can be distinguished by their mean ages (Fig. 5C,E,F): compounds that reach ages of ~80–280 years, i.e. centennial compounds (depicted in red in Fig. 5E), and compounds that reach ages of 6,000–11,000 years, i.e. millennial compounds (depicted in blue in Fig. 5E). The centennial compounds comprise those three compound groups that are supplied from external sources such as primary production. The millennial compounds comprise all other compounds, which are exclusively produced by microbes, at much lower rates. The modelled age spectrum thus emerges from differences in the supply rate of compounds (i.e. their rate of autotrophic production). Note that our simulated microbes do not distinguish between the centennial and millennial compounds during uptake or release. The uptake and release network is constructed randomly, without any preference for the two compound groups. A microbe in the model could be able to take up centennial along with millennial compounds, depending on the random outcome of the network. The bi-modal age distribution in the simulation (Fig. 5C,F) is consistent with observations⁷. The relative width of the two age peaks and their distinctiveness is set by the difference in the supply rate of the respective compounds.
根据平均年龄，可以区分两组化合物（图5C、E、F）：达到约80-280年的化合物，即百年化合物（图5E中以红色表示），以及达到6,000-11,000年的化合物，即千年化合物（图5E中以蓝色表示）。百年化合物包括那些从外部来源（如初级生产）供应的三个化合物组。千年化合物包括所有其他化合物，这些化合物仅由微生物以较低速率产生。因此，模拟的年龄谱是由化合物供应速率的差异（即它们的自养生产速率）产生的。请注意，我们模拟的微生物在摄取或释放过程中不区分百年和千年化合物。摄取和释放网络是随机构建的，没有对这两组化合物有任何偏好。模型中的微生物可能能够摄取百年和千年化合物，这取决于网络的随机结果。模拟中的双峰年龄分布（图5C、F）与观察结果一致。两个年龄峰的相对宽度和独特性由相应化合物的供应速率差异确定。

The more microbial reworking is needed to form a compound from algal-produced DOC, the older it becomes. In this sense, our model is in agreement with the processes termed the “microbial carbon pump”: DOC with high mean ages is produced by microbes⁴¹. The variation in ages within the two age classes is explained by the uptake and release within the network of compounds and degrading microbes. Within the microbial compounds (average age of ~8,000 years), for example, some compounds are relatively younger, showing ages of only ~6,000 years. Those compounds are direct release products of microheterotrophs which consume fresh, algal DOC. The age of a compound is thus a consequence of its supply rate and its position in the network of uptake and release preferences. This mechanism could explain observed DOC spectra additional to the traditional view that the observed age spectrum of DOC compounds arises from differences in intrinsic reactivity of DOC compounds⁴².
越需要微生物重组来形成藻类产生的DOC化合物，它就越老。从这个意义上说，我们的模型与被称为“微生物碳泵”的过程是一致的：高平均年龄的DOC是由微生物产生的。两个年龄类别内的年龄变化是通过化合物和降解微生物网络中的摄取和释放来解释的。例如，在微生物化合物中（平均年龄约为8,000年），一些化合物相对较年轻，只有约6,000年的年龄。这些化合物是微异养生物的直接释放产物，它们消耗新鲜的藻类DOC。化合物的年龄因此是其供应速率和在摄取和释放偏好网络中的位置的结果。这个机制可以解释观察到的DOC光谱，除了传统观点认为观察到的DOC化合物的年龄谱是由DOC化合物固有反应性的差异引起的。

Age fractions of observed marine DOC show distinct structural properties: Fresh DOC compounds are larger, have a lower C:N ratio, and a higher percentage of common biochemicals than aged DOC compounds^42,43. This co-occurrence of structural and age differences does not necessarily require the assumption of intrinsic compound stability. If structurally different compound classes differ in production rates, the structural signature will be reflected in the age fractions. Hence, if we assume a general decrease in size from highly-produced compounds (e.g. algal DOM) to low-produced compounds (e.g. hydrothermal DOM⁴⁴), then aged, modelled compounds will be smaller in molecular size than young compounds. Indeed, algal DOM has been shown to be relatively large, whereas hydrothermally altered DOM shows decreased molecular mass. Accordingly, the model results can be reconciled with the size-reactivity-continuum⁴³.
观测到的海洋DOC的年龄分数表现出明显的结构特性：新鲜的DOC化合物较大，C:N比例较低，常见生物化合物的百分比较高，而老化的DOC化合物则相反。这种结构和年龄差异的共存并不一定需要假设化合物本身稳定。如果结构不同的化合物类别在产生速率上有所不同，结构特征将反映在年龄分数中。因此，如果我们假设从高产化合物（例如藻类DOM）到低产化合物（例如热液DOM）的普遍尺寸减小，则老化的、建模的化合物比年轻的化合物分子要小。事实上，已经表明藻类DOM相对较大，而热液改变的DOM显示分子质量降低。据此，该模型的结果与大小-反应性-连续性可以得到调和。

Implications for DOC stability
DOC稳定性的影响

The mechanism behind the longevity of DOC in the model
模型中DOC长寿的机制

Although we assume bioavailability of all compounds, the model predicts long-lived fractions of DOC (Fig. 5). Usually, it is assumed that microbially-produced DOC in the ocean persists over millennia, because bacteria alter its structure, making it intrinsically stable^16,42 (see Supplement Fig. S7 for a comparison to Shen & Benner’s experiment). However, in our model, microheterotrophs cannot alter the reactivity of DOC compounds. Instead, microbes exclusively re-distribute the carbon among the compounds (through the network of uptake and release) and reduce DOC concentration (through respiration).
尽管我们假设所有化合物都具有生物可利用性，但模型预测DOC的长寿分数（图5）。通常，人们认为海洋中由微生物产生的DOC会持续数千年，因为细菌会改变其结构，使其本质上稳定（参见附图S7与Shen和Benner的实验进行比较）。然而，在我们的模型中，微异养生物无法改变DOC化合物的反应性。相反，微生物仅通过摄取和释放的网络在化合物之间重新分配碳，并通过呼吸减少DOC浓度。

Ultimately, the longevity of DOC in our model can be explained by the limitation of microbial growth by low DOC concentrations. This corresponds to the well-known resource competition theory of Tilman: Analogous to the R* value, that gives “the levels to which each competitor can reduce a single limiting resource”⁴⁰, we propose a D* value, the minimum DOC concentration that can be reached by biological degradation. For a scenario of neutrality, D* can be estimated from Eq. 1, otherwise the full network model is required. D* is reached when the growth of the microheterotrophs balances their mortality, and when the production of DOC (i.e. the sum of supply, release, and lysis) balances its biological degradation. In this steady-state, DOC concentrations and biomasses equilibrate, such that net changes become zero. This equilibrium DOC level does not allow for further microbial growth and biomass increase; hence DOC is (apparently) recalcitrant.
最终，我们模型中DOC的长寿可以通过低DOC浓度限制微生物生长来解释。这与蒂尔曼的资源竞争理论相对应：类似于R*值，它给出了“每个竞争者可以降低单一限制资源的水平”，我们提出了一个D*值，即可以通过生物降解达到的最低DOC浓度。对于中立情况的场景，可以从方程1估计D*，否则需要完整的网络模型。当微异养生物的生长平衡其死亡时，以及DOC的产生（即供应、释放和溶解的总和）平衡其生物降解时，D*被达到。在这个稳态中，DOC浓度和生物量达到平衡，净变化为零。这个平衡的DOC水平不允许进一步的微生物生长和生物量增加；因此DOC是（表面上）难降解的。

If the equilibrium DOC level D* is surpassed via supply of DOC compounds from an external source, the formerly recalcitrant DOC allows for microbial growth¹⁰ (see Supplement Fig. S8). Through this process, recalcitrant deep-sea DOC can be microbially removed near the ocean surface, as reported e.g. by Cherrier et al.⁴⁵ (the authors of this study suggest photodegradation causes this removal, here we offer an alternative explanation). According to the notation of Jiao et al.¹⁸, the equilibrium DOC level D* would correspond to the RDOC_c, defined as “compounds that may be inaccessible to microbes due to their extremely low concentration“. Assumptions about RDOC_t (“compounds that are resistant to microbial consumption in certain environments, but subject to further cleaving and decomposition when the situation changes”¹⁸) are not required to form a recalcitrant carbon pool at concentrations close to observed values for experiments and the deep sea in our model.
如果通过来自外部来源的DOC化合物的供应超过了平衡的DOC水平D*，以前难降解的DOC可以促进微生物生长（见补充图S8）。通过这个过程，表面接近海洋的深层海底DOC可以被微生物去除，例如Cherrier等人报道（本研究的作者指出光降解引起了这种去除，我们提供了另一种解释）。根据焦等人的符号表示，平衡的DOC水平D*将对应于RDOC，定义为“由于极低浓度可能无法被微生物接触的化合物”。我们的模型不需要对RDOC的假设（“在某些环境中对微生物消耗具有抵抗性的化合物，但在情况改变时可能进一步裂解和分解”）形成接近观察值的实验和深海的难降解碳池。

Intrinsic stability: An alternative model set-up
内在稳定性：另一种模型设置

Additional to our neutral model set-up, we implemented an alternative model set-up comprising the five traditional reactivity classes of DOC (labile, semi-labile, semi-refractory, refractory, and ultra-refractory⁴). In this alternative model set-up, intrinsic reactivity differences are implemented by successively reducing the maximum uptake rate by an order of magnitude for each less reactive DOC class (Supplementary material, Fig. S9). The supply of DOC is restricted to the labile DOC class, i.e. recalcitrant DOC is exclusively microbially-produced. In this alternative model set-up, DOC concentration does not reach a steady-state, but increases due to ongoing accumulation of the least reactive DOC classes (i.e. refractory and ultra-refractory DOC). Applied to the ocean, this would imply an increase of DOC concentrations with each ocean overturning. To achieve an equilibrium DOC level in this “intrinsic stability model”, additional abiotic removal process to microbial consumption would have to be postulated, e.g. adsorption to sinking particles or photodegradation in the sunlit ocean⁴.
除了我们的中性模型设置外，我们还实施了一个替代模型设置，包括DOC的五个传统反应性类别（易降解、半易降解、半难降解、难降解和超难降解）。在这个替代模型设置中，通过逐渐降低每个较不活性DOC类别的最大摄取速率一个数量级来实现内在的反应性差异（附录材料，图S9）。DOC的供应仅限于易降解的DOC类别，即难降解的DOC完全由微生物产生。在这个替代模型设置中，DOC浓度不会达到稳定状态，而是由于最不活性的DOC类别（即难降解和超难降解DOC）的持续积累而增加。应用于海洋，这将意味着每次海洋翻转都会增加DOC浓度。为了在这个“内在稳定模型”中实现平衡的DOC水平，还必须假设存在额外的非生物去除过程，例如吸附到下沉颗粒物或在阳光照射下的光降解。

Main findings 主要发现

We use a network model of DOC-microbe-interactions to predict long-term DOC behaviour. In our model all DOC compounds are equally bioavailable. Our numerical model is in agreement with observed characteristics of marine DOC (Table 1).
我们使用DOC-微生物相互作用的网络模型来预测长期DOC行为。在我们的模型中，所有的DOC化合物都是等同生物可利用的。我们的数值模型与海洋DOC的观测特征相一致（表1）。

In our model, a significant fraction of the bioavailable DOC resists microbial degradation, forming an apparently recalcitrant DOC pool. A fundamental microbiological principle prevents further degradation of DOC at low concentrations: microbial growth ceases when the concentration-dependent uptake of DOC balances the concentration-independent microbial carbon loss via mortality or maintenance, resulting in an equilibrium DOC level (D*). Consequently, the apparently recalcitrant DOC becomes available for microbial degradation, if concentrated. Under the assumption of complete neutrality, D* can be estimated through an extended version of the Michaelis-Menten concept where substrate diversity and microbial capabilities (n/n_U) play a central role (Eq. 1).
在我们的模型中，可生物利用的DOC的一部分抵抗微生物降解，形成一个表面上难降解的DOC池。一项基本的微生物学原则阻止了低浓度下DOC的进一步降解：当浓度依赖的DOC摄取平衡了浓度无关的微生物碳损失（通过死亡或维持）时，微生物生长停止，导致一个平衡的DOC水平（D*）。因此，如果浓缩，表面上难降解的DOC就变得可供微生物降解。在完全中立的假设下，可以通过扩展的Michaelis-Menten概念来估计D*，其中底物多样性和微生物能力（n/n _U ）起着核心作用（方程式1）。

We show that the size of the modelled DOC reservoir corresponds to observations in the deep ocean. Individual, equally bioavailable DOC compounds reach radiocarbon ages between modern and several thousand years. The age of DOC compounds in our model is not determined by intrinsic reactivity, but by their position in the network of microbial uptake and release, and, most importantly, by their rate of supply from primary production. Overall, our results indicate that the long-term stability of marine DOC emerges from basic physiological properties of the individual members of the degrading microbial community, and the resulting interactions between the individual microbial members through substrate units. As such, DOM stability is possibly neither a direct consequence of intrinsic recalcitrance, nor simply due to a fixed dilution threshold. The aim of this modelling study was not to prove or disprove the existence of intrinsically recalcitrant features in DOC, but to show that assuming neutral, concentration-limited uptake, a realistic size and age of the DOC reservoir can be reached.
我们展示了建模DOC储量的大小与深海观测相符。个体同等生物可利用DOC化合物的放射性碳年龄在现代和几千年之间。我们模型中DOC化合物的年龄不是由内在反应能力决定的，而是由它们在微生物摄取和释放网络中的位置，以及最重要的是它们从初级生产中的供应速率决定的。总体而言，我们的研究结果表明，海洋DOC的长期稳定性源于降解微生物群落成员的基本生理特性，并且通过底物单位之间的个体微生物成员之间的相互作用而形成。因此，DOM稳定性可能既不是内在难降解特性的直接结果，也不是简单地由固定的稀释阈值决定的。本模型研究的目的并非证明或反驳DOC中不易降解特性的存在，而是展示假设中性、浓度限制的摄取，可以达到合理的DOC储量大小和年龄。

Model equations 模型方程式

We consider m taxonomic units of microheterotrophs B_i (i = 1, 2, …, m) and n substrate units of DOC compounds D_j (j = 1, 2, …, n). Our model resolves the carbon concentration (in mmolC/m³) of these microbial units and DOC compounds in a mixed culture. Microbial units are measured in units of carbon to avoid stoichiometric conversion between the state variables. Modelled processes are summarized in Fig. 1. The model is composed of a set of ordinary differential equations, one for each individual microbial unit B_i and one for each DOC compound unit D_j (see Supplementary Material Equation (4) for the inorganic carbon pool I, equations (5–13) for the radiocarbon age, and Table S1 for a list of symbols and abbreviations):
我们考虑微异养体B（i = 1, 2, …, m）的m个分类单位和DOC化合物D（j = 1, 2, …, n）的n个底物单位。我们的模型解决了混合培养中这些微生物单位和DOC化合物的碳浓度（以mmolC/m为单位）。微生物单位的测量单位为碳，以避免状态变量之间的化学计量转换。建模过程总结在图1中。该模型由一组普通微分方程组成，每个微生物单位B和每个DOC化合物单位D都有一个方程（请参阅供补材料中方程（4）中的无机碳池I，方程（5-13）中的放射性碳年龄，以及符号和缩写列表）。

From the DOC uptake, a fraction η is fixed into microbial biomass, the fraction β(1 − η) is released back to the DOC pool as transformed compounds, and the remaining carbon is permanently transferred to the inorganic carbon pool (i.e. microbial respiration). DOC uptake is described according to the Michaelis-Menten kinetic, with the maximum uptake rate ρ and the half-saturation constant к. We assume that each substrate unit is concentration-limited individually. This means, if unit A is present at high concentrations and unit B at low concentrations, the former is taken up at high rates, while the uptake of the latter is concentration limited. Mortality of microbes is proportional to mortality rate μ and contributes to the formation of transformed DOC compounds. New DOC is supplied at the rate s from primary production (where the total supply rate s = ∑s_j is the sum of the supply rates for individual compounds s_j). The uptake matrix U = (U_ij) defines the uptake preferences of each microbial unit i, its entries U_ij specify whether a microbial unit can take up a compound unit j (U_ij > 0). The release matrix R = (R_ij) defines a specific set of release products for each microbial unit, as well as the partitioning of carbon among the release products. Its entries R_ij specify the fraction of carbon released per compound unit. Together, U and R form a “transformation network”, as the taken-up carbon is re-distributed among DOC compounds for release. Altogether, the total microbial biomass, the total DOC concentration, and the inorganic carbon concentration (ΣB_i, ΣD_j, I) form a mass-conserving carbon pool.
从DOC的摄取中，一部分η被固定为微生物生物量，一部分β(1-η)以转化化合物的形式释放回DOC池，剩余的碳则永久转移到无机碳池中（即微生物呼吸）。DOC的摄取根据Michaelis-Menten动力学描述，最大摄取速率为ρ，半饱和常数为к。我们假设每个底物单位都受浓度限制。这意味着，如果A单位的浓度很高，而B单位的浓度很低，前者的摄取速率很高，而后者的摄取受浓度限制。微生物的死亡率与死亡率μ成比例，并有助于形成转化的DOC化合物。新的DOC以速率s从初级生产中供应（其中总供应速率s = ∑s是各个化合物的供应速率之和）。摄取矩阵U = (U _ij )定义了每个微生物单位i的摄取偏好，其条目U _ij 指定了微生物单位是否可以摄取化合物单位j（U _ij > 0）。释放矩阵R = (R _ij )为每个微生物单位定义了一组特定的释放产物，以及碳在释放产物之间的分配。其条目R _ij 指定了每个化合物单位释放的碳的比例。U和R共同形成一个“转化网络”，因为摄取的碳在释放时重新分配给DOC化合物。 总的来说，总微生物生物量、总DOC浓度和无机碳浓度（ΣB，ΣD，I）形成一个质量守恒的碳库。

How the model works: an illustrative model set-up
模型的工作原理：一个形象的模型设置

To illustrate the basic model behaviour, we present a toy set-up, with a small number of microbial and compound units (Fig. 6). Initially, the DOC compound unit D₁ is provided as the only substrate (Fig. 6C). Initially, biomass of all microbial units is very low (Fig. 6D). The microbial unit B₁ takes up the initially provided compound unit D₁ (according to the uptake matrix in Fig. 6A, row 1, column 1), and transforms it into DOC compound units D₂ and D₃ (in a ratio of 9:1, according to the release matrix in Fig. 6B, row 1, column 2 and 3). The microbial units B₂ and B₃ start to grow on the newly produced compound units. B₃ reaches a higher maximum biomass than B₂, because it is able to grow on both compound units D₂ and D₃. The microbial unit B₃ produces the compound units D₄ and D₅, which serves as a substrate for the last blooming microbial unit B₄. This microbial unit releases carbon in the form of D₃ (40%) and the initially provided compound unit D₁ (60%), thereby closing the cycle of DOC re-working. The compound unit D₅ remains at concentrations near zero throughout the virtual incubation, because it is produced only at 20% by a single microbial unit (B₃), and it is taken up by all other three microbial units (B₁, B₂, B₄). Due to the respiration of organic carbon to inorganic carbon (time series not shown) the total concentration of DOC declines over time. There is no supply of DOC in this virtual incubation.
为了说明基本模型的行为，我们提供了一个玩具设置，其中包含少量的微生物和化合物单位（图6）。最初，只提供DOC化合物单位D ₁ 作为底物（图6C）。最初，所有微生物单位的生物量都非常低（图6D）。微生物单位B ₁ 吸收最初提供的化合物单位D ₁ （根据图6A中的摄取矩阵，第1行，第1列），并将其转化为DOC化合物单位D ₂ 和D ₃ （按照图6B中的释放矩阵，第1行，第2列和第3列的比例为9:1）。微生物单位B ₂ 和B ₃ 开始在新产生的化合物单位上生长。B ₃ 的最大生物量比B ₂ 高，因为它能够在化合物单位D ₂ 和D ₃ 上生长。微生物单位B ₃ 产生化合物单位D ₄ 和D ₅ ，这成为最后一个繁殖的微生物单位B ₄ 的底物。该微生物单位以D ₃ （40%）和最初提供的化合物单位D ₁ （60%）的形式释放碳，从而完成DOC再处理的循环。化合物单位D ₅ 在整个虚拟孵育过程中保持接近零的浓度，因为它只由一个微生物单位（B ₃ ）产生20%，并被其他三个微生物单位（B ₁ ，B ₂ ，B ₄ ）吸收。 由于有机碳呼吸为无机碳（时间序列未显示），DOC的总浓度随时间下降。在这个虚拟孵化过程中没有DOC的供应。

Illustration of the DOC decomposition model: a toy model set-up. The transformation network of DOC is defined by two matrices, (A) the uptake matrix U, and (B) the release matrix R. The model predicts (C) the concentration of individual DOC compound units D₁,…, D_n and (D) the biomass of each microbial unit B₁,…, B_m over time (here for simplicity only n = 5 and m = 4 groups are shown; see Supplement Fig. S10 for a corresponding representation with higher microbial and chemical diversity).
DOC分解模型的示意图：一个玩具模型设置。DOC的转化网络由两个矩阵定义，(A)吸收矩阵U和(B)释放矩阵R。该模型预测(C)各个DOC化合物单位D ₁ ，…，D _n 的浓度和(D)每个微生物单位B ₁ ，…，B _m 的生物量随时间的变化（这里仅显示了n = 5和m = 4组，为了简单起见，请参见补充图S10，其中显示了更高的微生物和化学多样性）。

Full size image

This simulated initial DOC decomposition qualitatively matches observations. It has been shown experimentally that a sequence of microbial groups with distinct functional and transporter profiles occur during decomposition of algal-derived organic matter; as the substrates become available successively and enable different ecological niches to be filled⁴⁶.
这种模拟的初始DOC分解与观察结果定性相符。实验证明，在藻类衍生有机物分解过程中，会出现一系列具有不同功能和转运蛋白谱的微生物群落序列；随着底物逐渐可利用，不同的生态位得以填充。

Default parameterization 默认参数化

The model parameters were chosen according to published reference values, if available (Table 2). Note that some of the parameters (e.g. the total number of compound units n, the half-saturation constant κ, and the number of compound units taken up per microbial unit n_U) are not well-constrained and the chosen parameter set has a high level of uncertainty. To account for this, we tested the sensitivity of steady-state total DOC, microbial biomass, and mean DOC age (see Fig. 3 and Supplement Figs. S2, S3) for each parameter. Microbial traits are assumed to be equal, apart from the uptake- and release abilities, i.e. all microbial units are assigned the same mortality rate, maximum uptake rate, half-saturation constant, and they take up and release the same number of compound units.
模型参数根据已发表的参考值进行选择（表2）。请注意，其中一些参数（例如化合物单位总数n、半饱和常数κ以及每个微生物单位吸收的化合物单位数n _U ）没有很好的限制，并且所选择的参数集存在较高的不确定性。为了考虑这一点，我们对稳态总DOC、微生物生物量和平均DOC年龄的敏感性进行了测试（见图3和补充图S2、S3）。微生物特性被假设为相等，除了吸收和释放能力外，即所有微生物单位被分配相同的死亡率、最大吸收速率、半饱和常数，并且它们吸收和释放相同数量的化合物单位。

Number of compounds, microbial units, substrates, and release products (n, m, n _U, n _R)
化合物、微生物单位、底物和释放产物的数量（n、m、n _U 、n _R ）

Marine microbes are highly diverse: Huber et al.⁴⁷ estimated the presence of >35,000 marine operational taxonomic units. The molecular diversity of DOC compounds is also high: Zark et al.⁴⁸ estimated that >100,000 different dissolved organic matter compounds exist in seawater. To increase computational efficiency, the diversity of both groups is reduced for numerical simulations. However, the diversity ratio (0.35: 1) is maintained by grouping marine microbes to a total of 35 microbial units, and DOC compounds to 100 compound units, respectively. Note that we define the compound units from a bacterial perspective: all compounds that can be taken up by the same physiological uptake mechanism (e.g. transporter proteins) are grouped together, i.e. in our model molecular structural details only matter if they are relevant in a physiological context. As published estimates are missing, we assume that a microbial unit can take up 3% of the compound units, and releases 30% of the compound units, reflecting the observation that organic matter is diversified by microbial degradation^31,49. Note that the absolute numbers of m, n, n_U, and n_R are not crucial in the context of the model, as long as their proportion and their relation with the uptake parameters is considered: the steady-state DOC concentration is independent of the number of microbial units (Fig. S5A), but is influenced strongly by the ratio n_U/n (Fig. S5B) and the half-saturation constant κ (Fig. S5C, see also discussion of size of DOC reservoir). For example, the diversity of DOC and microbes could be increased proportionally by an order of magnitude (m = 350, n = 1050, n_U = 30), however, this would only increase the size of the uptake- and release network, and leave the long-term DOC concentration D* unaffected (see Eq. (1)).
海洋微生物的多样性很高：Huber等人估计存在超过35,000个海洋操作分类单元。DOC化合物的分子多样性也很高：Zark等人估计海水中存在超过100,000种不同的溶解有机物化合物。为了提高计算效率，数值模拟中减少了两个群体的多样性。然而，通过将海洋微生物分组为总共35个微生物单元，将DOC化合物分组为100个化合物单元，保持了多样性比例（0.35:1）。请注意，我们从细菌的角度定义化合物单元：所有可以通过相同的生理摄取机制（例如转运蛋白）摄取的化合物被分组在一起，即在我们的模型中，分子结构细节只有在生理环境中相关时才重要。由于缺乏已发表的估计数据，我们假设一个微生物单元可以摄取3%的化合物单元，并释放30%的化合物单元，反映了有机物通过微生物降解而多样化的观察结果。请注意，在模型的背景下，m、n、n和n的绝对数量并不重要，只要考虑到它们与摄取参数的比例和关系：稳态DOC浓度与微生物单元的数量无关（图S5A），但受到n/n比例（图S5B）和半饱和常数κ（图S5C，还请参阅DOC储库大小的讨论）的强烈影响。 例如，DOC和微生物的多样性可以按数量级增加（m = 350，n = 1050，n _U = 30），然而，这只会增加摄取和释放网络的规模，并且不会影响长期DOC浓度D*（参见方程（1））。

Fractionation of uptake to biomass, release, and respiration (η, β)
吸收到生物量、释放和呼吸的分数化（η，β）

We assume that the amount of carbon taken up by a microbe is split across three different pathways: the fraction η is assigned to microbial growth, the fraction β(1 − η) is released as transformed DOC, and the remaining fraction (1 − η)(1 − β) is respired (i.e. the carbon is permanently assigned to the inorganic carbon pool). Estimates from experimental studies are available for microbial growth efficiency, which we use to choose η (BGE = 0.09–0.33^50,51). Note that productivity measurements are unlikely to record the released material, thus underestimating growth efficiency⁵⁰ and potentially biasing the estimate. The fraction of carbon uptake that is released β(1 − η) was chosen based on observations by Ogawa et al.³⁰, who found that 15% of taken up glucose was released as DOC after 2 days of incubation in experiments, and 13% for glutamate. The microbial growth efficiency could be reduced to account for additional energy required for the degradation of high molecular mass compounds via extracellular hydrolysis¹⁷. A reduction of the growth efficiency associated with the uptake of a fraction of DOC compounds by 10% would increase the long-term equilibrium concentration of this fraction by 10% (as given in Eq. (1)).
我们假设微生物吸收的碳量分配到三个不同的途径：分数η分配给微生物生长，分数β(1-η)释放为转化的DOC，剩余的分数(1-η)(1-β)被呼吸（即碳被永久分配给无机碳库）。实验研究提供了微生物生长效率的估计值，我们用它来选择η（BGE = 0.09-0.33）。注意，生产力测量不太可能记录释放的物质，因此低估了生长效率，并可能导致估计结果有偏差。选择释放的碳吸收比例β(1-η)是基于Ogawa等人的观察结果，他们发现在实验中，经过2天孵育后，15%的葡萄糖和13%的谷氨酸被释放为DOC。微生物生长效率可以降低，以考虑通过细胞外水解降解高分子量化合物所需的额外能量。生长效率的降低与吸收部分DOC化合物相关，降低10%将使该部分的长期平衡浓度增加10%（如公式（1）所示）。

Microbial uptake and mortality (ρ, κ, μ)
微生物的摄取和死亡（ρ，κ，μ）

Estimates for uptake parameters representative for the highly diverse microbial community of the global oceans are lacking to date, therefore uptake parameters were based on laboratory estimates of a facultatively oligotrophic marine ultramicrobacterium growing on either alanine or glucose in the absence of primary producers and grazers, yielding a maximum uptake rate ρ = 0.96–3.60 d⁻¹ and a half-saturation constant κ = 6–450 mmolC/m^3 52. The model results are qualitatively robust towards variation of these parameters by a factor of 0.5 to 1.5 (Fig. 3A for uptake rate, Fig. S4D for half-saturation constant). As the published estimates vary by nearly two orders of magnitude, the half-saturation constant κ is not well-constrained. Changes in the value of κ can be balanced by proportional changes in the ratio of compounds taken up per microbial unit to the total DOC diversity n_U/n (see Supplementary Figure S5). Note that this parameter choice likely overestimates microbial DOC consumption compared to oceanic conditions, yielding a conservative minimum estimate of the DOC reservoir size. It should also be noted that if uptake rates were assumed different among components, their individual long-term concentration would change accordingly, whereas the total DOC concentration would rather depend on the average over consumption rates. The mortality of microbes is assumed linearly proportional to their biomass, with a mortality rate μ = 0.02 d⁻¹. Laboratory experiments with planktonic microbes showed specific mortality rates of 0.009–0.025 d^−1 53 in the absence of grazers, similar to rates used in other modelling studies (0.031–0.05 d^−1 19,23).
迄今为止，缺乏代表全球海洋高度多样化微生物群落的摄取参数估计，因此摄取参数基于实验室估计的一种在无初级生产者和食草动物存在下生长的兼性寡营养海洋超微细菌，其最大摄取速率ρ = 0.96-3.60 d ⁻¹ ，半饱和常数κ = 6-450 mmolC/m ^{3 52} 。模型结果对这些参数的0.5到1.5倍变化具有定性的稳健性（摄取速率见图3A，半饱和常数见图S4D）。由于已发表的估计值变化接近两个数量级，半饱和常数κ并不确定。κ值的变化可以通过微生物单位摄取化合物与总DOC多样性n _U /n的比例变化来平衡（参见补充图S5）。请注意，与海洋条件相比，这种参数选择可能高估了微生物DOC消耗，从而得出了DOC储量的保守最小估计。还应注意，如果假设不同组分的摄取速率不同，它们各自的长期浓度将相应变化，而总DOC浓度将更多地取决于消耗速率的平均值。微生物的死亡率被假设与其生物量成线性比例关系，死亡率μ = 0.02 d ⁻¹ 。与浮游微生物的实验室实验显示出0.009-0.在没有放牧者的情况下，类似其他建模研究中使用的速率（0.031-0.05 d）持续。

Supply of DOC (s) DOC（s）的供应

Based on a labile DOC production in the ocean of 25 PgC/y = 5.71 × 10¹⁵ mmolC/d⁴, euphotic zone depth of 200 m, and global ocean surface area of 3.62 × 108 km^2 54, the total supply rate of DOC s in the surface layer is assumed to be 3.45 × 10⁻⁷ PgC km⁻³ y⁻¹ = 0.08 mmolC m⁻³ d⁻¹. Unless otherwise stated, the supply of DOC is distributed evenly across all DOC compound units (see Supplement Fig. S11 for the influence of supply diversity on the DOC concentration, microbial biomass, and mean DOC age). For the simulation of mean DOC age (Fig. 5), we restricted the supply of DOC to 3% of compounds, to reflect a scenario where phytoplankton releases a limited subset of DOC compounds, which are molecularly diversified during subsequent microbial degradation^31,32,49.
基于每年25 PgC的不稳定DOC产量，相当于5.71×10 ¹⁵ mmolC/d，在200米的光合带深度和全球海洋表面积3.62×10^8 km ^{2  54} 的条件下，假设表层DOC的总供应速率为3.45×10 ⁻⁷ PgC km ⁻³ y ⁻¹ ，相当于0.08 mmolC m ⁻³ d ⁻¹ 。除非另有说明，DOC的供应均均匀分布在所有DOC化合物单位上（参见补充图S11，了解供应多样性对DOC浓度、微生物生物量和平均DOC年龄的影响）。对于平均DOC年龄的模拟（图5），我们将DOC的供应限制在化合物的3%以内，以反映浮游植物释放出一部分有限的DOC化合物，并在随后的微生物降解过程中分子多样化 ^31,32,49 。

Uptake and release matrix (U, R)
摄取和释放矩阵（U，R）

The default uptake matrix U = (U_ij) is a binary matrix, where U_ij = 1 encodes that the DOC compound unit j is taken up by microbial unit i according to the maximum uptake rate; U_ij = 0 means that the DOC compound unit j is not taken up by microbial unit i. The matrix is randomly constructed in each model simulation with the following restrictions: i) all microbial units can take up the same number of substrates n_U, i.e. the sum over the rows is identical, ii) all DOC compound units are associated with a decomposer, i.e. each column has at least one entry U_ij = 1, and iii) there is no DOC compound unit which is consumed by all microbial units, i.e. each column has at least one entry U_ij = 0. The sum over the columns, is on average (m × n_U)/n = 1.05, however it differs slightly due to the random variations among the columns (standard variation of 0.23), thus each DOC compound unit has a slightly different total uptake rate.
默认的摄取矩阵U = (U _ij )是一个二进制矩阵，其中U _ij = 1表示微生物单元i按照最大摄取速率摄取DOC化合物单元j；U _ij = 0表示微生物单元i不摄取DOC化合物单元j。该矩阵在每个模型模拟中随机构建，具有以下限制：i）所有微生物单元可以摄取相同数量的底物n _U ，即行之和相同；ii）所有DOC化合物单元与分解者相关联，即每列至少有一个条目U _ij = 1；iii）没有任何DOC化合物单元被所有微生物单元消耗，即每列至少有一个条目U _ij = 0。列之和平均为(m × n _U )/n = 1.05，但由于列之间的随机变化而略有不同（标准差为0.23），因此每个DOC化合物单元的总摄取速率略有不同。

The default release matrix R = (R_ij) defines the partitioning of carbon among the release products, where R_ij = 0.3 means that the microbial unit i releases 30% of released carbon in the form of compound unit j. A fixed number of release products (n_R) per microbial unit is distributed randomly across the compound units. However, it is ensured that microbes do not release compound units they take up (i.e. R_ij = 0, if U_ij = 1). The row sums of the release matrix are equal to one, to preserve the mass balance of carbon. The sum over the columns differs due to random variations, thus each DOC compound unit has a slightly different total release rate (on average m/n = 0.35, with a standard deviation of 0.09).
默认的释放矩阵 R = (R _ij ) 定义了碳在释放产物中的分配，其中 R _ij = 0.3 表示微生物单元 i 以化合物单元 j 的形式释放的碳中有30％。每个微生物单元都以随机分布的方式分配一定数量的释放产物（n _R 个）给化合物单元。然而，确保微生物不会释放其所吸收的化合物单元（即，如果 U _ij = 1，则 R _ij = 0）。释放矩阵的行总和为1，以保持碳的质量平衡。由于随机变化，列的总和有所不同，因此每个 DOC 化合物单元的总释放速率略有不同（平均为 m/n = 0.35，标准偏差为0.09）。

The network of uptake and release abilities is constructed randomly. However, we show that the stochastic influence of the network on the long-term DOC concentration is low compared to the influence of the model parameters (see light green area in Fig. 3, indicating the variation between 50 randomly constructed networks). Similarly, Coles and colleagues⁵⁵ found indications that biogeochemical gradients of DOC are determined by the total available pool of metabolic functions, rather than by the distribution of functions among organisms.
摄取和释放能力的网络是随机构建的。然而，我们表明与模型参数的影响相比（参见图3中的浅绿色区域，表示50个随机构建网络之间的变化），网络对长期DOC浓度的随机影响较低。同样，Coles和同事发现，生物地球化学梯度的DOC是由可用的代谢功能总量决定的，而不是由功能在生物体之间的分布决定的。

Energy turnover of microbes
微生物的能量转化

To test the implications of the model parameterization on the assumed energy turnover of microbes, we calculated the energy gain from the complete oxidation of the DOC constituents for the individual microbial cells. The energy turnover of microbes is estimated assuming that the respiration in equilibrium matches the supply of DOC. According to LaRowe et al.⁵⁶, the standard molal Gibbs energy in the model is 0.0054 kJ/m³/d, assuming the default supply rate (s = 8 × 10⁻² mmolC/m³/d) and a mean nominal oxidation state of carbon of −0.2685 (based on 164 Atlantic DOM samples presented in Mentges et al.⁵⁷). For a mean cell number in the bathypelagic of 6 × 10¹⁰ (0.12 mmolC/m^3 35 at 25 fgC/cell⁵⁸), the energy turned over is 3.31 × 10⁻¹¹ kJ/cell/year. This is orders of magnitude higher than the basal power requirements of microheterotrophs, estimated for oxic and anoxic sediments (3.31 × 10⁻¹⁴ kJ/cell/year and 10⁻¹⁵ to 10⁻¹⁴ kJ/cell/year⁵⁹). Thus, in principle, microbial life in the deep sea can be sustained from the oxidation of DOC in a situation of dynamic steady-state⁷.
为了测试模型参数对微生物能量转化的影响，我们计算了从DOC组分完全氧化中微生物细胞获得的能量增益。假设微生物的能量转化是根据呼吸平衡与DOC供应相匹配进行估计的。根据LaRowe等人的研究，模型中的标准摩尔基比斯能是0.0054 kJ/m/d，假设默认的供应速率（s = 8 × 10 mmolC/m/d），以及碳的平均名义氧化状态为-0.2685（基于Mentges等人提供的164个大西洋DOM样本）。对于深海浴层中的平均细胞数量为6 × 10（以25 fgC/cell为基准），能量转化为3.31 × 10 kJ/cell/year。这比微型异养生物的基础功率需求高几个数量级，其估计值为氧化和无氧沉积物中的3.31 × 10 kJ/cell/year和10到10 kJ/cell/year。因此，原则上，深海中的微生物生命可以在动态稳态的DOC氧化情况下得到维持。

Simulating differential equations
模拟微分方程

The differential equations were solved numerically in MATLAB (Version 2017b, The MathWorks, Inc., Natick, Massachusetts, United States), using the non-stiff differential equation solver ode45, with the following options: maximum time step was 50 d, all state variables were set to be non-negative, and the relative error tolerance was set to 10⁻⁶. To prevent microbial biomass from dropping to the smallest positive normalized floating-point number in MATLAB (1 × 10⁻³⁰⁸) if no DOC is supplied, we added a constant influx of microbes at rate of 1 × 10⁻³⁰⁰ mmolC/m³/d. This corresponds to an inflow of roughly one microbial cell per day to a volume of 1 × 10²⁸⁸ m³ (carbon content of a single microbial cell ~2 × 10⁻¹² mmolC⁵⁸). As the volume of the ocean is about 1 × 10¹⁸ m³, this inflow of carbon is considered negligible relative to the state variables.
微分方程在MATLAB（版本2017b，The MathWorks，Inc.，马萨诸塞州纳蒂克，美国）中通过非刚性微分方程求解器ode45进行数值求解，使用以下选项：最大时间步长为50天，所有状态变量均设置为非负，相对误差容限设置为10 ⁻⁶ 。为了防止微生物生物量在没有提供DOC的情况下降至MATLAB中最小的正规化浮点数（1×10 ⁻³⁰⁸ ），我们添加了一个恒定的微生物流入速率为1×10 ⁻³⁰⁰ mmolC/m ³ /d。这相当于每天向1×10 ²⁸⁸ m ³ 的体积中流入大约一个微生物细胞（单个微生物细胞的碳含量约为2×10 ⁻¹² mmolC ⁵⁸ ）。由于海洋的体积约为1×10 ¹⁸ m ³ ，相对于状态变量来说，这种碳的流入被认为是可以忽略的。

Simulations 模拟

All simulations were initiated with a total biomass of 1 mmolC/m³ for the microbial community, representative for values typically observed in the surface ocean³⁵. At 25 fgC/cell⁵⁸, 1 mmolC/m³ of microbial biomass in the model translates to ~5 × 10¹¹ cells.
所有模拟都以微生物群落的总生物量为1 mmolC/m ³ 开始，这个值代表通常在海洋表层观察到的值 ³⁵ 。以每个细胞25 fgC的生物量计算，模型中的1 mmolC/m ³ 微生物生物量相当于约5 × 10 ¹¹ 个细胞。

Formation of a recalcitrant DOC pool
难降解的溶解有机碳（DOC）池的形成

The amount of (apparently) recalcitrant DOC was determined for three different supply-scenarios (Fig. 2): high supply rates of DOC (default eutrophic supply s = 8 × 10⁻² mmolC/m³/d), low supply rates of DOC (s = 8 × 10⁻³ mmolC/m³/d), and no supply of DOC (s = 0). The simulation was initiated with equal amounts of all DOC compound units and microbial units (in total 80 mmolC/m³ DOC, representing high surface-ocean values³⁴). The flux to the inorganic carbon pool in the high, low, and no supply scenarios was ~1, 3, and 30 mmolC/m³ per year on average.
在三种不同的供应情景下确定了（显然）难以处理的DOC的数量（图2）：高供应速率的DOC（默认富营养供应s = 8×10 ⁻² mmolC/m ³ /d），低供应速率的DOC（s = 8×10 ⁻³ mmolC/m ³ /d），以及没有DOC供应（s = 0）。模拟开始时，所有DOC化合物单位和微生物单位的数量相等（总共80 mmolC/m ³ DOC，代表高表面海洋值 ³⁴ ）。高、低和无供应情景下的向无机碳库的通量平均为约1、3和30 mmolC/m ³ 每年。

Size of the DOC reservoir: A sensitivity analysis
DOC储量的大小：敏感性分析

The steady-state DOC concentration was determined over a range of parameter values, based on 50 independent runs per parameter value (Fig. 3). Each parameter was varied individually, while the other parameters were kept at their default value. The parameters were varied between 50–150% of their default value. Note that the number of microbial units m could not be decreased by 50% without violating our basic assumption that every compound unit should be associated with a consuming microbial unit: at a default of 3 substrates per microbial unit and 100 compound units, at least 34 microbial units are required to maintain this basic assumption. Similarly, the number of compound units n could not be increased by 50%, and the number of substrates per microbe n_U could not be decreased by 50%.
基于每个参数值的50次独立运行，确定了稳态DOC浓度（图3）。每个参数都单独变化，而其他参数保持默认值。参数的变化范围为其默认值的50-150%。请注意，如果将微生物单位m减少50%，将违反我们的基本假设，即每个化合物单位应与一个消耗微生物单位相关联：在每个微生物单位3个底物和100个化合物单位的默认情况下，至少需要34个微生物单位来维持这个基本假设。同样，化合物单位n的数量不能增加50%，每个微生物的底物n也不能减少50%。

Millennial scale stability of DOC
千禧年尺度的DOC稳定性

The age of DOC compound units was simulated in a Lagrangian water parcel moving along the oceanic circulation (Fig. 4, for derivation of age see supplement). The water parcel was assumed to stay in near-surface layers for one year, subsequently sink to deeper waters, remain in the deep sea for 899 years (i.e. average circulation age of water in the Pacific⁶⁰), and finally rise back to the surface. The radiocarbon age is derived based on equations 4 through 12 in the supplement. The supply rate of DOC was variable over time to reflect the respective environmental conditions: high supply rates near the surface (default supply rate) and low supply rates of DOC in the deep sea from sinking particulate organic matter (one permil of the surface supply rate, as the global production of high molecular weight DOM in the deep ocean is estimated at 0.014 PgC/y⁵), which is ≈ 10⁻³ × 25 PgC/y surface labile DOC production⁴). The supply was linearly adapted to the new conditions after sinking/rising of the water parcel over a transition period of 10 days. To reflect the effect of an algal bloom, the supply of DOC was selectively distributed among the compounds, i.e. the total supply rate was split among a small, fixed subset of compound units. Note that we exclusively varied the DOC supply rate, whereas supply diversity was fixed throughout the simulation. The supply diversity was assumed at 3%, (see Supplement Fig. S11C for a sensitivity analysis of mean DOC age to this parameter). This setup reflects the observation that phytoplankton preferentially releases a limited subset of DOM compounds, which are molecularly diversified during subsequent microbial degradation^31,32,49. To quantify the degree to which a DOC compound unit is supplied versus microbially produced, we derived a measure of the “distance to supply” for each compound unit (Fig. 5D,E, note that the term distance is not used in sense used in the context of networks, i.e. number of edges between two points, but rather depicts a proportion). It was calculated based on the total fraction of carbon released by consumers of supplied compounds. This measure was inverted and rescaled, such that 0 indicates that a compound is directly supplied e.g. from algae, whereas 1 indicates that the compound is exclusively microbially produced.
DOC复合单位的年龄在一个拉格朗日水团中进行模拟，该水团沿着海洋环流移动（图4，有关年龄的推导请参见补充材料）。假设水团在近表层停留一年，随后下沉到更深的水域，在深海中停留899年（即太平洋水体的平均环流年龄 ⁶⁰ ），最后再次上升到表面。放射性碳年龄是根据补充材料中的方程4至12推导得出的。DOC的供应速率随时间变化，以反映相应的环境条件：表面附近的高供应速率（默认供应速率）和深海中由下沉的颗粒有机物贡献的低DOC供应速率（相当于表面供应速率的千分之一，因为深海中高分子量DOM的全球产量估计为0.014 PgC/y ⁵ ），这相当于10 ⁻³ × 25 PgC/y表面易耗性DOC产量 ⁴ ）。在水团下沉/上升后的过渡期间，供应速率线性适应新条件的变化，持续10天。为了反映藻华的影响，DOC的供应被选择性地分配给化合物单位，即总供应速率被分配给一个小的、固定的化合物单位子集。请注意，我们仅改变了DOC的供应速率，而供应多样性在整个模拟过程中保持不变。供应多样性被假设为3%（有关此参数对平均DOC年龄的敏感性分析，请参见补充材料图S11C）。 这种设置反映了浮游植物优先释放一部分有限的DOM化合物，这些化合物在随后的微生物降解过程中分子多样化。为了量化每个化合物单位的供应与微生物产生之间的程度，我们推导出了一个“供应距离”的度量（图5D、E，注意，这里的距离一词并非指网络中两点之间的边数，而是表示比例）。它是基于由供应化合物的消费者释放的碳的总分数计算的。这个度量被反转和重新缩放，使得0表示化合物直接从藻类等地方供应，而1表示该化合物完全由微生物产生。