Flow and Transport Properties of Deforming Porous Media. I. Permeability
变形多孔介质的流动和传输特性。I. 渗透率

Abstract
摘要

Estimating flow and transport properties of porous media that undergo deformation as a result of applying an external pressure or force is important to a wide variety of processes, ranging from injecting a fracking liquid into shale formations, to CO${}_2$ sequestration in spent oil reservoirs. We propose a novel model for estimating the effective flow and transport properties of such porous media. Assuming that the solid matrix of a porous medium undergoes elastic deformation, and given its initial porosity before deformation, as well as the Young’s modulus of its grains, the model uses an extension of the Hertz–Mindlin theory of contact between grains to compute the new PSD that results from applying an external pressure P to the medium, and utilizes the updated PSD in the effective-medium approximation (EMA) to estimate the effective flow and transport properties at pressure P. In the present part of this series, we use the theory to predict the effective permeability as a function of the applied pressure. Comparison between the predictions and experimental data for twenty-four types of sandstones indicates excellent agreement between the two.
估计由于施加外部压力或力而变形的多孔介质的流动和传输特性对于各种过程都至关重要，这些过程从向页岩层注入压裂液到在耗尽油藏中 CO2 ${}_2$ 储存。我们提出了一种新的模型来估计这种多孔介质的有效流动和传输特性。假设多孔介质的固体基质发生弹性变形，并给出其变形前的初始孔隙率以及其颗粒的杨氏模量，该模型使用 Hertz-Mindlin 颗粒接触理论的扩展来计算施加外部压力 P 到介质上产生的新 PSD，并利用更新的 PSD 在有效介质近似（EMA）中估计压力 P 下的有效流动和传输特性。在本系列的部分中，我们使用该理论预测施加压力作为有效渗透率函数。对二十四种砂岩的预测与实验数据的比较表明，两者之间有很好的吻合。

1 Introduction
1 引言

The focus of this paper is on the effect of deformation of porous media on their flow and transport properties, a problem that is encountered in a wide variety of contexts, and is of fundamental interest to geologists, reservoir engineers, and groundwater scientists. Examples include deformation of consolidating clays (Brown and Brindley 1980), geological formations deep underground where the pressure is large (Iliev et al. 2008; Fagbemi et al. 2018), geothermal, coal-bed methane, oil and gas reservoirs, as well as unconventional energy resources, such as shale formations. For example, to extract hot water from geothermal reservoirs at economically attractive rates, their porosity and permeability must be such that the volume flow rate of water is on the order of tens of m${}^3$/hr or larger (Heiland 2003). Often though, such reservoirs have low permeability and, therefore, they need stimulation by, for example, hydraulic fracturing in order to produce hot water economically.
本文重点关注多孔介质变形对其流动和传输特性的影响，这是一个在各种情况下都会遇到的问题，对地质学家、储层工程师和地下水科学家具有根本性的兴趣。例如，包括固结粘土的变形（Brown 和 Brindley 1980 年）、地下深处的大型地质构造（Iliev 等人 2008 年；Fagbemi 等人 2018 年）、地热、煤层甲烷、油气储层，以及非常规能源资源，如页岩层。例如，为了以具有经济吸引力的速度从地热储层中提取热水，其孔隙率和渗透率必须使得水的体积流量达到数十立方米/小时或更高（Heiland 2003 年）。然而，通常这些储层的渗透率较低，因此需要通过例如水力压裂等方式进行刺激，以便经济地生产热水。

Aside from laboratory experiments on the effect of deformation on the effective permeability (Zoback and Byerlee 1975), one also needs a predictive model that can provide accurate estimates for the pressure- or stress-dependence of the effective flow and transport properties. Although the problem has been studied experimentally by numerous groups (see, for example, Zhu and Wong 1997; Keaney et al. 1998; Ruisten et al. 1999; Ngwenya et al. 2003; Fossein et al. 2007; Baud et al. 2012; Ballas et al. 2015; Liu et al. 2018; Meng et al. 2019; Yang and Hu 2020), an accurate model for predicting the pressure-dependence of flow and transport properties of deforming porous media is still lacking.
除了对变形对有效渗透率（Zoback 和 Byerlee 1975）影响的实验室实验外，还需要一个可以提供对有效流动和传输性质的压力或应力依赖性准确估计的预测模型。尽管该问题已被多个研究小组通过实验进行研究（例如，参见 Zhu 和 Wong 1997；Keaney 等人 1998；Ruisten 等人 1999；Ngwenya 等人 2003；Fossein 等人 2007；Baud 等人 2012；Ballas 等人 2015；Liu 等人 2018；Meng 等人 2019；Yang 和 Hu 2020），但仍然缺乏一个准确预测变形多孔介质流动和传输性质压力依赖性的模型。

A related phenomenon is deformation of shale formations (Ibanez and Kronenberg 1993) under compression and varying confining pressure. When shale gas accumulates in rock, the pressure rises significantly as a result of the action of high-pressure gas, coupled with ground stress in the seam, implying that the surrounding rock is always under high confining stress. Due to their low porosity and permeability, shale formations will not produce, unless they are subject to hydraulic fracturing that generates a fracture network, with the induced fractures intersecting the natural ones (Osborn et al. 2011). After producing for a while, gas production decreases, leading to repeated fracturing. At the end, the pressures of the shale gas and the fracturing fluid both decrease, as the high-pressure fracturing fluid is discharged. Therefore, during the entire process, shale formations are subject to cyclic loading and water pressure, hence giving rise to stress–permeability coupling (Jiang et al. 2018).
相关现象是页岩层在压缩和不同围压下的变形（Ibanez 和 Kronenberg 1993）。当页岩气在岩石中积累时，由于高压气的作用以及层理中的地应力，压力显著上升，这意味着周围岩石始终处于高压围压之下。由于它们的低孔隙率和渗透率，页岩层不会产生气体，除非它们受到水力压裂，从而产生裂缝网络，诱导裂缝与自然裂缝相交（Osborn 等人，2011）。经过一段时间生产后，气体产量下降，导致重复压裂。最后，随着高压压裂液的排放，页岩气和压裂液的压强都下降。因此，在整个过程中，页岩层受到循环载荷和水的压力，从而产生应力-渗透率耦合（Jiang 等人，2018）。

Deformation of coal-bed methane reservoirs represents another example in which the permeability of the formations varies under uniaxial or triaxial stress. Several groups have developed theoretical models for the effect of an external load on the porosity and permeability of coals (see, for example, Liu and Harpalani 2013; Wu et al. 2018; Mathias et al. 2019). The cleats in coal formations contribute most to their permeability. Reducing the pore pressure increases the effective stress, leading to a reduction in the apertures of the coal cleats and, hence, a reduction in the porosity and permeability.
煤田甲烷储层变形是另一个例子，其中在单轴或三轴应力下，地层的渗透率会发生变化。几个研究小组已经开发了关于外部载荷对煤孔隙率和渗透率影响的理论模型（例如，参见 Liu 和 Harpalani 2013；Wu 等人 2018；Mathias 等人 2019）。煤层的裂隙对其渗透率贡献最大。降低孔隙压力会增加有效应力，从而导致煤裂隙开口减小，从而降低孔隙率和渗透率。

Deformation of solid materials is not, however, confined to rock, as there is a wide class of deformable porous materials whose flow and transport properties, when they are subject to an external load, vary with the magnitude of the load - pressure or stress. They range anywhere from polymers and hydrogels (Iritani et al. 2006; Karada 2010; Sweijen et al. 2017), the “cake” that is built up on the external surface of filters (Sahimi and Imdakm 1991; Imdakm and Sahimi 1987, 1991), and printing papers (Ghassemzadeh et al. 2001; Ghassemzadeh and Sahimi 2004; Masoudi and Pillai 2010), to diapers (Savoji and Pourjavadi 2006; Salimi et al. 2010), and foams (Koehler et al. 2000; Pitois et al. 2009). Another important example of much current interest is deformation and swelling of geological formations, such as oil and gas reservoirs that no longer produce, as a result of injecting CO${}_2$ into their pore space. As recent molecular dynamics simulations indicated (Rahromostaqim and Sahimi 2018, 2019), in the presence of brine, CO${}_2$ causes swelling of pure and mixed clays that may eventually lead to earthquakes (Maxwell et al. 2008; Tafti et al. 2013; Rother et al. 2013; Lee et al. 2016).
固体材料的变形不仅限于岩石，因为存在一大类可变形多孔材料，当它们受到外部载荷时，其流动和传输特性会随着载荷的大小（压力或应力）而变化。这些材料包括聚合物和亲水凝胶（Iritani 等人，2006；Karada，2010；Sweijen 等人，2017），过滤器外部表面形成的“饼”（Sahimi 和 Imdakm，1991；Imdakm 和 Sahimi，1987，1991），印刷纸张（Ghassemzadeh 等人，2001；Ghassemzadeh 和 Sahimi，2004；Masoudi 和 Pillai，2010），尿布（Savoji 和 Pourjavadi，2006；Salimi 等人，2010），以及泡沫（Koehler 等人，2000；Pitois 等人，2009）。另一个当前备受关注的重要例子是地质构造的变形和膨胀，例如由于向其孔隙空间注入 CO ${}_2$ 而不再生产的油气藏。如最近的分子动力学模拟所示（Rahromostaqim 和 Sahimi，2018，2019），在存在卤水的情况下，CO ${}_2$ 会导致纯和混合粘土膨胀，最终可能导致地震（Maxwell 等人，2008；Tafti 等人，2013；Rother 等人，2013；Lee 等人）。 2016).

The physical properties of porous media depend strongly on their morphology, namely, their pore shape, pore-size distribution (PSD), and pore connectivity. Deformation changes the morphology and, therefore, the macroscopic properties. Therefore, a main goal of studying deformation of porous media is predicting such properties as a function of the driving force for deformation, such as an external pressure or stress. In particular, one is interested in understanding the relation between the effective flow and transport properties of deforming porous media, which is typically smaller than the initial properties before deformation, and the external pressure; see for example, the aforementioned references, as well as Khoei and Mohammadnejad (2011), Bhandari et al. (2015), and Pesavento et al. (2017).
多孔介质的物理性质强烈依赖于其形态，即孔隙形状、孔隙尺寸分布（PSD）和孔隙连通性。变形会改变形态，从而改变宏观性质。因此，研究多孔介质变形的主要目标之一是预测变形驱动力（如外部压力或应力）作为函数的这些性质。特别是，人们感兴趣的是了解变形多孔介质的有效流动和传输性质与外部压力之间的关系，这种关系通常小于变形前的初始性质；例如，参见上述参考文献，以及 Khoei 和 Mohammadnejad（2011）、Bhandari 等人（2015）和 Pesavento 等人（2017）。

The foundation for modeling of flow and transport in deforming porous medium is provided by the momentum balance and the equations that govern elastic deformation of solids, together with mass balance and appropriate constitutive and state equations. In a macroscopic approach to the problem, one averages the microscopic conservation laws over a suitable volume of a porous formation and supplements the theory with empirical or semi-empirical constitutive relations for flow, transport and mechanical properties. Biot (1941, 1956) pioneered this approach, which has also been extended in order to study the same phenomena involving nonlinear material behavior (see, for example, Zienkiewicz and Shiomi 1994; Zienkiewicz et al. 1990; Schrefler et al. 1998; Li et al. 2004; Zhang et al. 2009), as well as when the pore space is only partially saturated by a fluid in the presence of a second fluid (see, for example, Khoei and Mohammadnejad 2011; Pesavento et al. 2017).
变形多孔介质中流动和传输建模的基础是由动量平衡和描述固体弹性变形的方程提供的，以及质量平衡和适当的本构和状态方程。在宏观方法处理该问题时，通过对孔隙形成中适当体积的微观守恒定律进行平均，并用经验或半经验的本构关系补充理论，以描述流动、传输和力学性质。Biot（1941，1956）开创了这种方法，该方法也被扩展以研究涉及非线性材料行为的相同现象（例如，参见 Zienkiewicz 和 Shiomi 1994；Zienkiewicz 等人 1990；Schrefler 等人 1998；Li 等人 2004；Zhang 等人 2009），以及当孔隙空间仅部分被第二流体饱和时（例如，参见 Khoei 和 Mohammadnejad 2011；Pesavento 等人 2017）。

Another macroscopic approach is the so-called mixture theory (see, for example, Atkin and Craine 1976a, b; Bowen 1982; Murad and Cushman 1996; Huyghe and Janssen 1997; Cowin and Cardoso 2012; Huyghe et al. 2017), which was derived by averaging the microscopic equations of mass, momentum and energy over a suitable length scale. The entropy inequality that describes the direction of dissipation of energy due to deformation was not, however, invoked for deriving the macroscale equations. As a result, the relationships that link macroscopic thermodynamic variables to the properties of porous media could not be derived directly. Hassanizadeh and Gray (1979a, b, 1990) combined the two aforementioned macroscopic approaches together with the entropy inequality in order to derive a generalization of Darcy’s law for the flow field in a deformable porous medium (see also Weinstein et al. 2008; Zhu et al. 2010).
另一种宏观方法是所谓的混合理论（例如，参见 Atkin 和 Craine 1976a, b；Bowen 1982；Murad 和 Cushman 1996；Huyghe 和 Janssen 1997；Cowin 和 Cardoso 2012；Huyghe 等人 2017），该理论通过对适当长度尺度上的质量、动量和能量微观方程进行平均而得出。然而，描述由于变形而导致的能量耗散方向的熵不等式并未用于推导宏观尺度方程。因此，将宏观热力学变量与多孔介质性质联系起来的关系无法直接得出。Hassanizadeh 和 Gray（1979a, b, 1990）将上述两种宏观方法与熵不等式相结合，以推导出适用于可变形多孔介质中流动场的 Darcy 定律的推广（也可参见 Weinstein 等人 2008；Zhu 等人 2010）。

Since flow and transport in heterogeneous porous media are controlled by their morphology, a few computational approaches have also been developed that carry out numerical simulations, either in the image of porous media, or in a model of them (see, for example, Zhu and Wong 1999; Boutt and McPherson 2002; Arns et al. 2001, 2002; Dautriat et al. 2009; Thovert and Adler 2011; Jasinski et al. 2015; Bakhshian and Sahimi 2016; Bakhshian et al. 2018; Fagbemi et al. 2018; Aljasmi and Sahimi 2020) in order to simulate the changes in the morphology of porous media as they undergo deformation.
由于异质多孔介质中的流动和传输受其形态控制，因此也开发了一些计算方法，通过数值模拟，在多孔介质的图像或模型中进行，以模拟多孔介质在变形过程中形态的变化（例如，参见 Zhu 和 Wong 1999；Boutt 和 McPherson 2002；Arns 等 2001，2002；Dautriat 等 2009；Thovert 和 Adler 2011；Jasinski 等 2015；Bakhshian 和 Sahimi 2016；Bakhshian 等 2018；Fagbemi 等 2018；Aljasmi 和 Sahimi 2020）。

The goal of our study is developing a relatively simple, and to our knowledge, new, theoretical model for predicting the effective flow and transport properties of porous media that deform under an external hydrostatic pressure P. The model that we develop combines a theory of deformation of contacting grains under an external force with the effective-medium approximation (EMA) in order to predict the effective flow and transport properties as a function of P. Bruggeman (1935) was the first to develop an EMA for estimating the macroscopic properties of heterogeneous media. The same formulation was developed independently by Landauer (1952) for computing electrical conductivity of composite solids. The EMA was used by Koplik et al. (1984) to predict the effective permeability and conductivity of a porous medium.
我们的研究目标是开发一个相对简单、据我们所知，新的理论模型，用于预测在外部静水压力 P 作用下变形的多孔介质的有效流动和传输特性。我们开发的模型将接触颗粒在外力作用下的变形理论与有效介质近似（EMA）相结合，以预测 P 作为函数的有效流动和传输特性。Bruggeman（1935）首次开发了一个 EMA 来估计异质介质的宏观特性。Landauer（1952）独立地开发了相同的公式来计算复合固体的电导率。Koplik 等人（1984）使用 EMA 来预测多孔介质的有效渗透率和电导率。

In addition, other theories have been suggested for predicting the permeability. For example, Wadsworth et al. (2016) proposed a universal scaling of the permeability, which is based on the power laws of percolation theory. But all the past works, including those that utilized the EMA, were for predicting physical properties of rigid heterogeneous media (for comprehensive reviews see Sahimi 2003, 2011). What we present is, however, the application of a novel combination of the EMA and a theory of deformation for computing the macroscopic permeability and electrical conductivity of porous media that undergo deformation, when a hydrostatic pressure P is applied to them. In the present paper, Part I of a series, we develop the essential theory and utilize it to predict the effective permeability. In subsequent parts, the theory will be utilized for predicting the effective electrical conductivity of fluid-saturated porous media and other properties.
此外，还提出了其他理论来预测渗透率。例如，Wadsworth 等人（2016 年）提出了一种渗透率的通用尺度，该尺度基于渗流理论的幂律。但所有过去的工作，包括那些利用 EMA 的工作，都是为了预测刚性行异质介质的物理性质（参见 Sahimi 2003，2011 年的全面综述）。然而，我们所提出的是 EMA 与变形理论的一种新颖组合的应用，用于计算在施加静水压力 P 时发生变形的多孔介质的宏观渗透率和电导率。在本文中，这是一系列论文的第一部分，我们发展了基本理论并利用它来预测有效渗透率。在随后的部分中，该理论将被用于预测流体饱和多孔介质的有效电导率和其他性质。

The organization of this paper is as follows. In Sect.2, we derive the new theoretical model for estimating the change in the size of the pores when the pressure P is applied to a porous medium. The theory is then used in Sect. 3 to determine the evolution of the PSD during deformation. Section 4 describes the EMA for estimating the effective permeability. The proposed theory involves the elastic modulus of the grains or the solid matrix, and, therefore, Sect. 5 describes the various approaches for estimating the modulus. The computational procedure is described in Sect. 6, while Sect. 7 presents the theoretical predictions for the permeability of a wide variety of sandstones and compares them with experimental data. Section 8 discusses a few aspects of the model, while the paper is summarized in Sect. 9.
本文组织如下。在第 2 节中，我们推导了在压力 P 作用于多孔介质时估计孔隙尺寸变化的新理论模型。然后在第 3 节中，利用该理论确定变形过程中 PSD 的演变。第 4 节描述了用于估计有效渗透率的 EMA。所提出的理论涉及颗粒或固体基质的弹性模量，因此第 5 节描述了估计模量的各种方法。第 6 节描述了计算过程，而第 7 节提出了各种砂岩的渗透率的理论预测，并将它们与实验数据进行了比较。第 8 节讨论了模型的一些方面，而第 9 节总结了全文。

2 Theory of Elastic Deformation of Porous Media Under an Applied Force
孔隙介质在受力下的弹性变形理论

As mentioned in the Introduction, we employ the EMA to predict the effective permeability of porous media that deform as a result of applying an external pressure or stress. The EMA is a sort of mean-field approximation (MFA) that replaces a heterogeneous porous medium by an effective one in which all the pores have the same effective size $r_e$. The solution of the flow and transport problem in the uniform system is straightforward. Then, one pore in the effective medium is selected at random and its radius in the original disordered medium is restored, with the rest of the pores still having the same size $r_e$. This generates a perturbation in the solution of the uniform system, whose magnitude is the difference between the solution for the uniform medium and one that is uniform everywhere, but in one pore. The perturbation is calculated, and since the single pore is selected at random and its size follows a PSD, in order to be able to still represent the disordered medium with one with an effective pore radius $r_e$ and an effective flow or transport property, one insists that the average of the perturbation, when the averaging is taken over the PSD, should be zero. In effect, only the interaction of a single pore with the rest of the effective medium is taken into account, and the influence of the remaining part of the disordered porous medium is represented by the far-field external pressure gradient. Extensions of the EMA that account for interactions of a pore with those that are farther than the nearest-neighbor ones were also developed (Sahimi et al. 1983, 1984), but we ignore them in this work.
如引言所述，我们采用 EMA 来预测由于施加外部压力或应力而变形的多孔介质的有效渗透率。EMA 是一种平均场近似（MFA），它用一个所有孔隙具有相同有效尺寸的有效多孔介质来代替异质多孔介质。在均匀系统中解决流动和传输问题是直接的。然后，在有效介质中随机选择一个孔隙，并在原始无序介质中恢复其半径，其余孔隙保持相同尺寸。这会在均匀系统的解中产生扰动，其大小是均匀介质解与在单个孔隙中均匀的解之间的差异。计算扰动，由于单个孔隙是随机选择的，其尺寸遵循 PSD，为了仍然能够用一个具有有效孔隙半径和有效流动或传输特性的介质来表示无序介质，坚持在 PSD 上取扰动的平均值应为零。 实际上，只考虑单个孔隙与有效介质其余部分的相互作用，而将无序多孔介质其余部分的影响表示为远场外部压力梯度。还开发了考虑孔隙与比最近邻孔隙更远的孔隙相互作用的扩展 EMA（Sahimi 等，1983，1984），但我们在本研究中忽略了它们。

We assume that the deformation is elastic. At very high pressures, the inelastic effects may become important, but the range of pressure over which the experimental data that we use with the theoretical predictions is in the range of elastic deformation. Thus, in the spirit of the EMA, and for the sake of developing a tractable theoretical approach that is consistent with the mean-field nature of the EMA, we consider the interaction between two grains, the minimum number of grains in a MFA, subject to an external force F or the corresponding hydrostatic pressure P applied to the medium, and determine the deformation that it causes in the pore between the two grains that changes its size. The effect of the deformation of the rest of a porous medium is represented by the far-field applied pressure P or force. The pressure P changes the PSD of the pore space that, in turn, influences its effective flow and transport properties, and in particular its effective permeability $K_e$. Since one important input to the EMA is the PSD of the pore space, the first step toward the goal of predicting the dependence of $K_e$ on the external pressure P is to determine the changes in the PSD as P is gradually increased.
我们假设变形是弹性的。在非常高的压力下，非弹性效应可能变得重要，但我们所使用的实验数据与理论预测的压力范围在弹性变形范围内。因此，本着 EMA 的精神，为了发展一个与 EMA 的平均场性质一致的可处理的理论方法，我们考虑两个颗粒之间的相互作用，这是 MFA 中颗粒的最小数量，受到外部力 F 或施加到介质上的相应静水压力 P 的作用，并确定它引起的变形，这种变形改变了两个颗粒之间的孔隙大小。孔隙空间的有效流动和传输特性，特别是其有效渗透率 $K_e$ ，由远场施加的压力 P 或力表示。压力 P 改变了孔隙空间的 PSD，反过来又影响了其有效流动和传输特性。 由于 EMA 的一个重要输入是孔隙空间的 PSD，预测 $K_e$ 对外部压力 P 的依赖关系的首要步骤是确定 PSD 随 P 逐渐增加时的变化。

To determine the interaction between two neighboring grains and the deformation of the pore space between them, we derive a theory of deformation of contacting grains, first studied by Hertz (1882) who assumed no friction between the grains. Goodman (1962) studied the same problem, but with friction between the two grains, while (Mindlin 1949) considered the case in which tangential forces and twisting were coupled at the contact point between two grains. The derivation that we present is similar to the analysis of Timonshenko and Goodier (1970), but we present the complete details, simplify the analysis, and our final results were not actually presented by them.
为了确定两个相邻颗粒之间的相互作用以及它们之间孔隙空间的变形，我们推导了一个接触颗粒的变形理论，这一理论首先由赫兹（1882 年）研究，他假设颗粒之间没有摩擦。古德曼（1962 年）研究了相同的问题，但考虑了两个颗粒之间存在摩擦的情况，而（Mindlin 1949 年）考虑了在两个颗粒接触点处切向力和扭转力耦合的情况。我们提出的推导与 Timonshenko 和 Goodier（1970 年）的分析类似，但我们提供了完整的细节，简化了分析，并且我们的最终结果实际上并没有被他们提出。

Fig. 1
图 1

a Configuration of two grains and the pore between them for estimating the new pore radius. b The loaded zone for computing the pressure distribution, with point A being on the contact surface
a 两个颗粒及其之间的孔隙配置，用于估算新的孔隙半径。b 计算压力分布的加载区域，点 A 位于接触表面

We consider a typical, or average, grain size $R_g$, and normalize all the length scale with respect to it. Consider, then, Fig. 1a that shows two grains, 1 and 2, which, in the absence of any applied external pressure, are in contact at point C along the Z axis. We assume that the grains’ surfaces at the point of contact have radii of curvature $R_1$ and $R_2$. If the grains are roughly spherical, then, $R_1$ and $R_2$ also represent roughly their radii. Consider the plane tangent at C, and two points A and B that are on the front sections of the surfaces 1 and 2 at a small distance x from the Z axis. The distances $z_1$ and $z_2$ of A and B from the tangent plane satisfy the relations, $(R_1-z_1{)}^2+x^2=R_1^2$, and $(R_2-z_2{)}^2+x^2=R_2^2$. Assuming that $z_1$ and $z_2$ are small enough that $z_1^2$ and $z_2^2$ can be ignored, we obtain, $z_1=x^2/(2R_1)$ and $z_2=x^2/(2R_2)$. Therefore, the distance $D=z_1+z_2$ between A and B is given by
我们考虑一个典型或平均的颗粒尺寸 $R_g$ ，并将所有长度尺度相对于它进行归一化。考虑图 1a，它显示了两个颗粒 1 和 2，在没有施加任何外部压力的情况下，它们在 Z 轴上的点 C 处接触。我们假设颗粒在接触点的表面曲率半径为 $R_1$ 和 $R_2$ 。如果颗粒大致呈球形，那么 $R_1$ 和 $R_2$ 也大致代表它们的半径。考虑 C 点的切平面，以及位于表面 1 和 2 的前部，距离 Z 轴一小段距离 x 的点 A 和 B。点 A 和 B 到切平面的距离 $z_1$ 和 $z_2$ 满足关系 $(R_1-z_1{)}^2+x^2=R_1^2$ 和 $(R_2-z_2{)}^2+x^2=R_2^2$ 。假设 $z_1$ 和 $z_2$ 足够小，以至于 $z_1^2$ 和 $z_2^2$ 可以忽略，我们得到 $z_1=x^2/(2R_1)$ 和 $z_2=x^2/(2R_2)$ 。因此，点 A 和 B 之间的距离 $D=z_1+z_2$ 由以下公式给出：

$$\begin{array}{r}D=\left(\frac{R_1+R_2}{2R_1{R}_2}\right)x^2.\end{array}$$

(1)

An external force F is applied to the porous medium to press the grains together, which causes local deformation near C over a small, roughly circular (spherical) surface, which we refer to as the contact surface (CS). If the deformation is small, we may assume that both $R_1$ and $R_2$ are much larger than the radius of the CS. Suppose that $u_A$ and $u_B$ are, respectively, the displacements of A and B due to the local deformation along the Z axis. Then, if the tangent plane at C is held fixed, the local compression causes any two points on the surface of the two grains, which are far from C, to move toward each other by an amount u, implying that the distance between A and B decreases by $u-(u_A+u_B)$ and, therefore, the effective pore radius between the two grains also decreases by u/2. Thus, if the compression caused by applying the force F brings A and B into the CS, we must have,
一个外力 F 作用于多孔介质，将颗粒压紧，导致 C 点附近的小、大致圆形（球形）表面上发生局部变形，我们称之为接触面（CS）。如果变形很小，我们可以假设 $R_1$ 和 $R_2$ 都远大于 CS 的半径。假设 $u_A$ 和 $u_B$ 分别是 A 和 B 沿 Z 轴方向由于局部变形产生的位移。那么，如果保持 C 点的切平面固定，局部压缩会使两个颗粒表面远离 C 的两个点相互移动一个量 u，这意味着 A 和 B 之间的距离减少 $u-(u_A+u_B)$ ，因此，两个颗粒之间的有效孔隙半径也减少 u/2。因此，如果施加力 F 引起的压缩将 A 和 B 带入 CS，我们必须有，

$$\begin{array}{r}u-(u_A+u_B)=\alpha x^2,\mathrm{w}\mathrm{i}\mathrm{t}\mathrm{h}\alpha =\frac{R_1+R_2}{2R_1{R}_2},\end{array}$$

(2)

if we use Eq. (1). Thus,
如果我们使用公式（1）。因此，

$$\begin{array}{r}u=u_A+u_B+\alpha x^2.\end{array}$$

(3)

Therefore, we must determine $u_A$ and $u_B$ in order to compute u, i.e., the decrease in the distance between non-contacting surfaces of two grains, which leads directly to the change in the size of the pore between them and, hence, the change in the PSD of the deforming porous medium can be determined.
因此，我们必须确定 $u_A$ 和 $u_B$ ，以便计算 u，即两个颗粒非接触表面之间距离的减小，这直接导致它们之间孔隙大小的变化，从而可以确定变形多孔介质的 PSD 变化。

Consider a small element of the loaded zone, shown by the shaded area in Fig. 1b that is bounded between radii s and $s+\text{d}s$ and angle $\text{d}\theta$ where, as shown in Fig. 1a, A is a point on the CS. If p is the local pressure in the CS, then, the displacement $u_A$ is given by (Timoshenko and Goodier 1970),
考虑加载区域的一个小元素，如图 1b 中阴影所示，该元素被半径 s 和 $s+\text{d}s$ 以及角度 $\text{d}\theta$ 所界定，如图 1a 所示，A 是 CS 上的一个点。如果 p 是 CS 中的局部压力，那么，位移 $u_A$ 由（Timoshenko 和 Goodier 1970）给出，

$$\begin{array}{r}{u}_A=\left(\frac{1-{\nu }_1^2}{\pi E_1}\right)\int \int p\text{d}s\text{d}\theta ,\end{array}$$

(4)

which follows directly from the theory of displacement of a spherical grain, where $E_1$ and ${\nu }_1$ are, respectively, the elastic (Young’s) modulus and Poisson’s ratio of the grain. A similar equation also holds for $u_B$:
根据球形颗粒位移理论直接得出，其中 $E_1$ 和 ${\nu }_1$ 分别为颗粒的弹性（杨氏）模量和泊松比。类似的方程也适用于 $u_B$ ：

$$\begin{array}{r}{u}_B=\left(\frac{1-{\nu }_2^2}{\pi E_2}\right)\int \int p\text{d}s\text{d}\theta .\end{array}$$

(5)

Note that the two equations for $u_A$ and $u_B$ are subject to the condition that both A and B are on the CS. Therefore, by substituting the expressions for $u_A$ and $u_B$ in Eq. (3), we obtain
请注意， $u_A$ 和 $u_B$ 这两个方程满足条件 A 和 B 都在 CS 上。因此，通过将 $u_A$ 和 $u_B$ 的表达式代入方程（3），我们得到

$$\begin{array}{r}\frac{1}{\pi }(\frac{1-{\nu }_1^2}{E_1}+\frac{1-{\nu }_2^2}{E_2})\int \int p\text{d}s\text{d}\theta =u-\alpha x^2.\end{array}$$

(6)

It remains to calculate p, the local pressure distribution over the CS. If a hemisphere of radius $R_c$ is constructed on the CS, then building on the Hertz–Mindlin work, we argue that the pressure distribution is represented by the hemisphere’s ordinates. This implies that the pressure $p_0$ at the center of the CS, i.e., the maximum pressure in the CS, is simply proportional to $R_c$ and is given by, $p_0=a{R}_c$, where a is a scale factor. As shown in Fig. 1b, the local pressure p varies over a chord mn, shown by the dashed semicircle. Therefore, $\int pds=p_{0}S/R_c$, with S being the area of the semicircle. Since
它还需要计算 p，即 CS 上的局部压力分布。如果在 CS 上构建一个半径为 $R_c$ 的半球，那么基于 Hertz-Mindlin 的工作，我们认为压力分布由半球的纵坐标表示。这意味着 CS 中心的压力 $p_0$ ，即 CS 中的最大压力，简单地与 $R_c$ 成正比，并由 $p_0=a{R}_c$ 给出，其中 a 是一个尺度因子。如图 1b 所示，局部压力 p 在弦 mn 上变化，由虚线半圆表示。因此， $\int pds=p_{0}S/R_c$ ，其中 S 是半圆的面积。由于

$$\begin{array}{r}S=\frac{1}{2}\pi (R_c^2-x^2{\sin }^2\theta ),\end{array}$$

(7)

then, substituting for $\int pds$ and S in Eq. (6), yields
然后，将 、 $\int pds$ 和 S 代入方程（6），得到

$$\begin{array}{r}\left(\frac{p_0}{R_c}\right)(\frac{1-{\nu }_1^2}{E_1}+\frac{1-{\nu }_2^2}{E_2}){\int }_0^{\pi /2}(R_c^2-x^2{\sin }^2\theta )\text{d}\theta =u-\alpha x^2.\end{array}$$

(8)

Carrying out the integration, we obtain
进行积分，我们得到

$$\begin{array}{r}\left(\frac{\pi p_0}{4R_c}\right)(2R_c^2-x^2)(\frac{1-{\nu }_1^2}{E_1}+\frac{1-{\nu }_2^2}{E_2})=u-\alpha x^2.\end{array}$$

(9)

Equation (9) is an identity in terms of x that must be valid for any of its values. This would be possible if
方程（9）是一个关于 x 的恒等式，对于其任何值都必须成立。如果

$$\begin{array}{rl}& R_c=\left(\frac{\pi p_0}{4\alpha }\right)(\frac{1-{\nu }_1^2}{E_1}+\frac{1-{\nu }_2^2}{E_2}),\end{array}$$

(10)

$$\begin{array}{rl}& u=\left(\frac{1}{2}\pi p_0{R}_c\right)(\frac{1-{\nu }_1^2}{E_1}+\frac{1-{\nu }_2^2}{E_2}).\end{array}$$

(11)

To relate $p_0$ to the applied force F, we note that the sum of the pressures in the contact area multiplied by its surface should be equal to F. Thus, $(p_0/R_c)(\frac{2}{3}\pi R_c^3)=F$, or, $p_0=3F/(2\pi R_c^2)$, which, after substituting in Eq. (10) and solving for $R_c$, yields,
为了将 $p_0$ 与施加的力 F 联系起来，我们注意到接触面积内压力的总和乘以其表面积应等于 F。因此， $(p_0/R_c)(\frac{2}{3}\pi R_c^3)=F$ ，或者， $p_0=3F/(2\pi R_c^2)$ ，将其代入方程(10)并解出 $R_c$ ，得到，

$$\begin{array}{r}{R}_c={\left[\frac{3F}{8\alpha }(\frac{1-{\nu }_1^2}{E_1}+\frac{1-{\nu }_2^2}{E_2})\right]}^{1/3}.\end{array}$$

(12)

If we substitute Eq. (12) and the result for $p_0$ in Eq. (11), we find that
如果我们用方程（12）和方程（11）中 $p_0$ 的结果来替换，我们发现

$$\begin{array}{r}u=\frac{1}{2}{\left[3F(\frac{1-{\nu }_1^2}{E_1}+\frac{1-{\nu }_2^2}{E_2}){\left(\frac{R_1+R_2}{2R_1{R}_2}\right)}^{1/2}\right]}^{2/3}.\end{array}$$

(13)

Assuming that the two grains are composed of the same materials, we have, ${\nu }_1={\nu }_2=\nu$ and $E_1=E_2=E_e$. We also assume that the two grains have roughly the same radii of curvature, $R_1\approx R_2=R$. With the assumption of local isotropy, the local force is isotropic and homothetic, i.e., it is a monotonic transformation of F. Thus, F and the hydrostatic pressure P are related by, $F=\sqrt{2}{R}_g^{2}P=\sqrt{2}P$, with the second equation being due to normalization of lengths by $R_g$ (i.e., $R_g\to R_g/R_g=1$), where $\sqrt{2}$ is due to the geometrical considerations, as shown by Deresiewicz (1958). Under these conditions, Eqs. (12) and (13) are simplified to
假设两种颗粒由相同材料组成，我们有， ${\nu }_1={\nu }_2=\nu$ 和 $E_1=E_2=E_e$ 。我们还假设两种颗粒具有大致相同的曲率半径， $R_1\approx R_2=R$ 。在局部各向同性的假设下，局部力是各向同性和相似性的，即它是 F 的单调变换。因此，F 和静水压力 P 之间的关系为， $F=\sqrt{2}{R}_g^{2}P=\sqrt{2}P$ ，第二个方程是由于通过 $R_g$ （即， $R_g\to R_g/R_g=1$ ）归一化长度而产生的，其中 $\sqrt{2}$ 是由于几何考虑，如 Deresiewicz（1958）所示。在这些条件下，方程（12）和（13）简化为

$$\begin{array}{rl}& R_c=(R{)}^{1/3}{\left[\frac{3\sqrt{2}P(1-{\nu }^2)}{4E_e}\right]}^{1/3},\end{array}$$

(14)

$$\begin{array}{rl}& u={\left(\frac{1}{R}\right)}^{1/3}{\left[\frac{3P(1-{\nu }^2)}{E_e}\right]}^{2/3}.\end{array}$$

(15)

Thus, writing u and R in un-normalized units, Eqs. (14) and (15) become,
因此，将 u 和 R 用未归一化单位表示，方程（14）和（15）变为：

$$\begin{array}{rl}& R_c=R_g{\left(\frac{R}{R_g}\right)}^{1/3}{\left[\frac{3\sqrt{2}P(1-{\nu }^2)}{4E_e}\right]}^{1/3},\end{array}$$

(16)

$$\begin{array}{rl}& u=R_g{\left(\frac{R_g}{R}\right)}^{1/3}{\left[\frac{3P(1-{\nu }^2)}{E_e}\right]}^{2/3}.\end{array}$$

(17)

Note that, $R_g\approx R$, if the grains are roughly spherical, which we assume to be the case or, at the minimum, we can define a radius for an equivalent spherical particle.
请注意， $R_g\approx R$ ，如果颗粒大致呈球形，我们假设这种情况成立，或者至少我们可以为等效球形颗粒定义一个半径。

3 Evolution of the Pore-Size Distribution Under an External Hydrostatic Pressure
孔隙尺寸分布在外部静水压力下的演变

Within the framework of a MFA in which the interactions of two neighboring grains with other grains farther away are ignored, the pore between the two grains does not also interact with the pores farther away. Thus, as pointed out earlier, to a first-order approximation, the effective radius of a pore under an external hydrostatic pressure P decreases by u/2, where u is given by Eq. (17). In other words, the initial PSD distribution $f_0(r_0)$ before any pressure is applied is transformed to a new PSD $f_P(r_P)$ at pressure P where, $r_P=r_0-u/2$. If $f_0(r_0)$ is given, either analytically or numerically, then, since, $f_P(r_P)=f_0(r_0-u/2)$, one either has an analytical expression for $f_P(r_P)$, or constructs it numerically for any pressure P.
在忽略两个相邻颗粒与其他较远颗粒相互作用的 MFA 框架内，两个颗粒之间的孔隙也不与较远的孔隙相互作用。因此，如前所述，在第一阶近似下，孔隙在外部静水压力 P 下的有效半径减少 u/2，其中 u 由公式（17）给出。换句话说，在施加任何压力之前，初始 PSD 分布 $f_0(r_0)$ 转换为压力 P 下的新 PSD 分布 $f_P(r_P)$ ，其中 $r_P=r_0-u/2$ 。如果 $f_0(r_0)$ 已给出，无论是解析还是数值，那么，由于 $f_P(r_P)=f_0(r_0-u/2)$ ，对于任何压力 P，我们要么有 $f_P(r_P)$ 的解析表达式，要么数值构建它。

4 The EMA for the Effective Permeability
4 有效渗透率的 EMA

A porous medium consists of pore throats connected together at the pore bodies. The effective sizes of both the pore throats and pore bodies are distributed according to statistical distributions $f_t(r_t)$ and $f_b(r_b)$. It is, however, not straightforward to measure $f_b(r_b)$, which is why it is usually not available. Thus, since the macroscopic permeability is controlled by the pore throats, for convenience we refer to the pore throats as pores, and their distribution f(r) as the PSD. As described in Sect. 2, in the EMA a heterogeneous pore space is represented by a uniform medium with the size of all the pores being $r_e$. We assume that the pores are cylindrical. Then, for slow flow the flow conductance $K_f$ is given by, $K_f\propto r^4$ (other pore shapes may also be considered). Note that it is possible to consider other pore shapes. The EMA predicts that the macroscopic permeability $K_e$ is given by (Doyen 1988; David et al. 1990)
多孔介质由相互连接的孔隙喉部和孔隙体组成。孔隙喉部和孔隙体的有效尺寸均按统计分布 $f_t(r_t)$ 和 $f_b(r_b)$ 分布。然而，测量 $f_b(r_b)$ 并不简单，这也是它通常不可用的原因。因此，由于宏观渗透率受孔隙喉部控制，为了方便起见，我们将孔隙喉部称为孔隙，并将它们的分布 f(r)称为 PSD。如第 2 节所述，在 EMA 中，非均匀孔隙空间由所有孔隙尺寸为 $r_e$ 的均匀介质表示。我们假设孔隙是圆柱形的。然后，对于慢速流动，流动导纳 $K_f$ 由 $K_f\propto r^4$ 给出（也可以考虑其他孔隙形状）。请注意，可以考虑其他孔隙形状。EMA 预测宏观渗透率 $K_e$ 由（Doyen 1988；David 等 1990）给出。

$$\begin{array}{r}{K}_e=\frac{\varphi }{C_s\tau }\frac{r_e^4}{⟨r_b^2⟩},\end{array}$$

(18)

with $\varphi$ being the porosity, $\tau$ is the flow tortuosity for which various theories, as well as empirical and semi-empirical relations have been developed (for a review see Ghanbarian-Alavijeh et al. 2013), $C_s$ is a geometrical factor with $C_s=8$ for cylindrical pores in Hagen–Poiseuille (slow or laminar) flow, and $r_b$ is the size of the pore bodies. Since the distribution $f_b(r_b)$ of the size of the pore bodies is typically not available, David et al. (1990) suggested that one should use, $⟨r_b^2⟩\simeq ⟨r^2⟩={\int }_{r_m}^{r_M}{r}^{2}f(r)\text{d}r$, with $r_m$ and $r_M$ being, respectively, the minimum and maximum pore radii; we do the same in this paper. $r_e^4$ is computed by the EMA:
$\varphi$ 为孔隙率， $\tau$ 是流动曲折度，针对这一参数已发展出各种理论以及经验公式和半经验公式（参见 Ghanbarian-Alavijeh 等，2013 年的综述）， $C_s$ 是一个几何因子， $C_s=8$ 用于 Hagen–Poiseuille（慢速或层流）流动中的圆柱形孔隙， $r_b$ 是孔隙体的尺寸。由于孔隙体尺寸的分布 $f_b(r_b)$ 通常不可用，David 等（1990 年）建议使用， $⟨r_b^2⟩\simeq ⟨r^2⟩={\int }_{r_m}^{r_M}{r}^{2}f(r)\text{d}r$ ，其中 $r_m$ 和 $r_M$ 分别是孔隙的最小半径和最大半径；本文也采用同样的方法。 $r_e^4$ 由 EMA 计算得出。

$$\begin{array}{r}{\int }_{r_m}^{r_M}\frac{r_e^4-r^4}{r^4+(D-1)r_e^4}f(r)\text{d}r=0,\end{array}$$

(19)

Here, D is the Euclidean dimensionality of the porous medium ($D=3$ in our calculations). If the porosity of the porous medium is low enough that the pore space is near its critical porosity ${\varphi }_c$ or the percolation threshold, i.e., the porosity at which the sample-spanning cluster of the pores is barely connected, and the macroscopic permeability and electrical conductivity vanish for $\varphi \le {\varphi }_c$, then, as first derived by Kirkpatrick (1971), one may use Z/2 in Eq. (19), instead of D, where Z is the mean connectivity of the pore space.
这里，D 是多孔介质的欧几里得维度（在我们的计算中为 $D=3$ ）。如果多孔介质的孔隙率足够低，以至于孔隙空间接近其临界孔隙率 ${\varphi }_c$ 或渗透阈值，即孔隙的样本跨度簇几乎相连的孔隙率，并且宏观渗透率和电导率在 $\varphi \le {\varphi }_c$ 时消失，那么，如 Kirkpatrick（1971）首先导出的，可以使用 Z/2 替代 D，其中 Z 是孔隙空间的平均连通性。

Mukhopadhyay and Sahimi (2000) derived an EMA for predicting the direction-dependent macroscopic permeabilities of anisotropic porous media; Stroud (1975) presented a continuum EMA for anisotropic media in which the local conductivity or permeability was a tensor; Ghanbarian et al. (2016) utilized Eq. (19) to predict the relative permeability of water in soil in the presence of air; Ghanbarian and Javadpour (2017) invoked Eq. (19) to estimate the gas permeability in shales, while saturation-dependent electrical conductivity of partially saturated packings of spherical particles was computed by Ghanbarian and Sahimi (2017) using Eq. (19).
Mukhopadhyay 和 Sahimi（2000）推导了一个 EMA 来预测各向异性多孔介质的方向相关宏观渗透率；Stroud（1975）提出了一种适用于各向异性介质的连续 EMA，其中局部电导率或渗透率是一个张量；Ghanbarian 等人（2016）利用公式（19）预测了存在空气时土壤中水的相对渗透率；Ghanbarian 和 Javadpour（2017）调用公式（19）估计页岩中的气体渗透率，而 Ghanbarian 和 Sahimi（2017）使用公式（19）计算了球形颗粒部分饱和堆积的饱和度相关电导率。

5 Estimating the Parameters of the Model
估计模型的参数

Let us first point out that the model presented in Sect. 4 is a MFA. Therefore, similar to any MFA, the fluctuations in the local properties are ignored, allowing one to analyze the behavior of the system based on only two grains, the minimum number for a meaningful analysis. Similar to all the MFAs, the approach has its limitations and strengths, which we will discuss in Sect. 8. For now, it suffices to mention that since this is a two-grain MFA and, as a result, only an average grain size is required. We will return to this point in Sect. 8.
首先让我们指出，第 4 节中提出的模型是一个 MFA。因此，与任何 MFA 一样，局部属性的波动被忽略，允许人们仅基于两个颗粒来分析系统的行为，这是有意义的分析所需的最小颗粒数。与所有 MFAs 一样，这种方法有其局限性和优点，我们将在第 8 节中讨论。现在，只需提到，由于这是一个双颗粒 MFA，因此只需要平均颗粒尺寸。我们将在第 8 节中回到这一点。

5.1 The Poisson’s Ratio and the Pore-Size Distribution
5.1 膨胀系数和孔隙率分布

According to Eqs. (17) and (19), the parameters of the model are the Young’s modulus $E_e$, the Poisson’s ratio $\nu$, and the PSD f(r). If experimental data are available for the three parameters, they can be used directly in the theory. Unfortunately, for the sandstones that we analyze, the information is not available. Thus, we need to make judicious choice of the parameters. Our preliminary computations indicated that while the predictions of the model are sensitive to the value of the Young’s modulus, they only change mildly when $\nu$ and the PSD are varied, which we now demonstrate.
根据（17）式和（19）式，模型参数为杨氏模量 $E_e$ 、泊松比 $\nu$ 以及 PSD f(r)。如果对于这三个参数有实验数据，可以直接用于理论中。不幸的是，对于我们分析的砂岩，这些信息并不可用。因此，我们需要谨慎选择参数。我们的初步计算表明，虽然模型的预测对杨氏模量的值很敏感，但当 $\nu$ 和 PSD 变化时，它们的变化很小，我们现在将证明这一点。

Consider, first, the sensitivity of the predictions to the PSD. To study this, we used the following theoretical PSD distribution,
首先，考虑预测对 PSD 的敏感性。为了研究这一点，我们使用了以下理论 PSD 分布，

$$\begin{array}{r}f(r)=\left[\frac{r-r_m}{(r_0-r_m{)}^2}\right]\exp [-\frac{1}{2}{\left(\frac{r-r_m}{r_0-r_m}\right)}^2],\end{array}$$

(20)

Fig. 2
图 2

a The PSD distributions generated by Eq. (20) with $r_m$ held constant at $0.18\mu$m. b The PSD that was utilized in most of the computations, which was reported by Lindquist et al. (2000). The units of r are $\mu$m
a. 通过保持 $0.18\mu$ m 不变，由公式(20)生成的 PSD 分布。b. 在大多数计算中使用的 PSD，由 Lindquist 等人（2000 年）报道。r 的单位是 $\mu$ m。

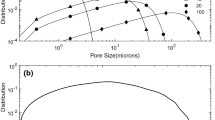
where $r_0$ is a parameter related to the average pore size $r_a$ through, $r_a=(r_0-r_m)\sqrt{\pi /2}+r_m$. We fixed the minimum pore size $r_m$ at $0.18\mu$m, the lowest pore sizes that we could identify in the published PSDs for sandstones, and varied $r_0$ over two orders of magnitude. Figure 2a presents the type of the PSD that Eq. (20) generates. The distribution generated by the lowest $r_0$ in Fig. 2 has striking similarities with what was reported by Fredrich et al. (1993) for a Fontainebleau sandstone, while those generated by other values of $r_0$ are qualitatively similar to those reported by others for other types of sandstone. We then computed the effective permeability for one of the sandstones that we analyze later in this paper, namely, the Tensleep sandstone (see Sect. 7), fixing all the parameters, but varying the PSD.
式中， $r_0$ 是与平均孔径 $r_a$ 通过， $r_a=(r_0-r_m)\sqrt{\pi /2}+r_m$ 相关的参数。我们将最小孔径 $r_m$ 固定为 $0.18\mu$ m，这是我们在发表的沙岩 PSD 中能识别出的最低孔径，并在两个数量级内变化 $r_0$ 。图 2a 展示了由方程（20）生成的 PSD 类型。图 2 中最低 $r_0$ 生成的分布与 Fredrich 等人（1993 年）报道的 Fontainebleau 沙岩非常相似，而其他 $r_0$ 值生成的分布与其他人报道的其他类型沙岩的分布定性相似。然后，我们计算了本文后面分析的一种沙岩（即 Tensleep 沙岩，见第 7 节）的有效渗透率，固定所有参数，但变化 PSD。

Figure 3 presents the results, where the permeability is normalized by its value before deformation (see also Sect. 7). The results do not indicate great sensitivity to the PSD. Calculations for all the sandstones that we analyzed (see Sect. 7) indicated the same trends. Thus, in the absence of any experimental data for the PSDs of the sandstones that we analyzer below, we used in all the cases described below the distribution presented in Fig. 2b as the initial PSD, $f_0(r_0)$, which was reported by Lindquist et al. (2000) for a Fontainebleau sandstone, and is similar to those for many other sandstones reported by others (see, for example, Cheung et al. 2012 for Bleurswiller and Boise sandstones). Note that the distribution is also similar to what Eq. (20) generates, and that the pore sizes vary over about two orders of magnitude, a relatively broad range.
图 3 展示了结果，其中渗透率已按其变形前的值进行归一化（参见第 7 节）。结果表明对颗粒尺寸分布（PSD）不敏感。对我们所分析的所有砂岩的计算（参见第 7 节）都表明了相同的变化趋势。因此，在缺乏我们下面分析的砂岩 PSD 的任何实验数据的情况下，我们使用图 2b 中所示的分布作为初始 PSD， $f_0(r_0)$ ，这是 Lindquist 等人（2000 年）为 Fontainebleau 砂岩报道的，并且与其他许多其他人报道的砂岩相似（例如，参见 Cheung 等人 2012 年关于 Bleurswiller 和 Boise 砂岩的报道）。请注意，该分布也与方程（20）生成的分布相似，并且孔径在约两个数量级内变化，范围相对较广。

Fig. 3
图 3

Effect of the PSD on the predicted permeabilities. $r_0$ is the parameter related to the average pore size $r_a$ (see the text), while $r_m=0.16\mu$m
PSD 对预测渗透率的影响。 $r_0$ 是与平均孔径 $r_a$ 相关的参数（见正文），而 $r_m=0.16\mu$ m

Next, we studied the sensitivity of the predictions to the value of the Poisson’s ratio, $\nu$. Once again, all the parameters but $\nu$ were fixed, and the model was used to predict the dependence on the applied pressure of the permeability of the sandstone of Fig. 3. Figure 4 presents the results. The Poisson’s ratio was varied by a factor of 4, and yet the predictions vary by at most 2 percent. Calculations with all the other sandstones that we analyze below (see Sect. 7) indicated the same trends. Thus, we fixed the Poisson’s ratio at $\nu \approx 0.3$, which is in the middle of the range for sandstones.
接下来，我们研究了预测对泊松比值的敏感性， $\nu$ 。再次，除了 $\nu$ 之外的所有参数都被固定，并使用该模型预测图 3 中砂岩的渗透率随施加压力的变化。图 4 展示了结果。泊松比以 4 倍变化，但预测值的变化最多仅为 2%。对我们下面分析的所有其他砂岩的计算（见第 7 节）也显示了相同趋势。因此，我们将泊松比固定在 $\nu \approx 0.3$ ，这是砂岩范围内的中间值。

Fig. 4
图 4

Effect of the Poisson’s ratio $\nu$ on the predicted permeabilities
泊松比 $\nu$ 对预测渗透率的影响

5.2 The Young’s Modulus of the Grains
5.2 粒子的杨氏模量

Let us first emphasize that the Young’s modulus $E_e$ in Eq. (17) is not that of the porous medium as a whole, which depends on its porosity and for which an EMA has been developed (Makse et al. 2001), but rather it is that of the grains, or the solid matrix of the porous medium in the MFA, which should either be measured, estimated theoretically, or is treated as an adjustable parameter. The grains are, however, hardly pure materials; they usually represent composites of several components. If the composition of the solid matrix or grains is known, then there are at least two theoretical approaches that can be used to estimate $E_e$.
让我们首先强调，方程（17）中的杨氏模量 $E_e$ 并不是整个多孔介质的杨氏模量，它取决于其孔隙率，并且已经开发了一种 EMA（Makse 等人，2001 年），而是指颗粒的杨氏模量，或者说是多孔介质在 MFA 中的固体基质，这应该要么进行测量，要么从理论上估计，或者作为可调整的参数处理。然而，颗粒几乎不是纯材料；它们通常代表由几个成分组成的复合材料。如果已知固体基质或颗粒的成分，那么至少有两种理论方法可以用来估计 $E_e$ 。

One method of estimating the elastic moduli of the solid matrix is through rigorous upper and lower bounds. Over the years, relatively tight bounds have been derived that provide reasonable estimates of the elastic moduli of solid composites. These are described in detail by Torquato (2002) and Sahimi (2003), to whom the interested reader is referred. The second approach is based on the so-called self-consistent approximation (SCA) for the effective elastic moduli of a composite material, first developed by Budiansky (1965), Hill (1965), and Wu (1966), and developed further by Berryman (1980), which is the analog of the EMA for the elastic moduli. For example, if we assume that the solid matrix is composed of two components, say quartz and clay as in many sandstones, such that the spatial distribution of clay (component 1) with volume fraction ${\varphi }_1$ is represented by identical spheres dispersed in the background matrix made of quartz—component 2 with volume fraction ${\varphi }_2$—then, according to the SCA the effective bulk modulus $B_e$ and shear modulus ${\mu }_e$ of the matrix are the solution of the following nonlinear coupled equations:
一种估计固体基质的弹性模量的方法是通过对严格的上界和下界进行估计。多年来，已经得到了相对紧密的界限，这些界限可以提供对固体复合材料的弹性模量的合理估计。这些内容在 Torquato（2002）和 Sahimi（2003）的详细描述中，有兴趣的读者可以参考。第二种方法是基于所谓的自洽近似（SCA）来估计复合材料的有效弹性模量，该方法最初由 Budiansky（1965）、Hill（1965）和 Wu（1966）开发，并由 Berryman（1980）进一步发展，它是弹性模量的 EMA 的类似物。例如，如果我们假设固体基质由两个成分组成，比如石英和粘土，就像许多砂岩中那样，那么粘土（成分 1）的体积分数为 ${\varphi }_1$ 的空间分布由在由石英（成分 2）组成的背景基质中分散的相同球体表示——体积分数为 ${\varphi }_2$ ——那么，根据 SCA，基质的有效体积模量 $B_e$ 和剪切模量 ${\mu }_e$ 是以下非线性耦合方程的解：

$$\begin{array}{r}{\varphi }_1\frac{B_e-B_1}{4{\mu }_e/3+B_1}+{\varphi }_2\frac{B_e-B_2}{4{\mu }_e/3+B_2}=0,\end{array}$$

(21)

and
并且

$$\begin{array}{r}{\varphi }_1\frac{{\mu }_e-{\mu }_1}{C_e{\mu }_e+{\mu }_1}+{\varphi }_2\frac{{\mu }_e-{\mu }_2}{C_e{\mu }_e+{\mu }_2}=0,\end{array}$$

(22)

where $C_e=(9B_e+8{\mu }_e)/(6B_e+12{\mu }_e)$. The case in which component 1 is spatially distributed in the background matrix as elliptical particles has also been studied (Berrymnan 1980). Equation (21) and (22) are accurate, provided that ${\varphi }_1\ll {\varphi }_2$.
其中 $C_e=(9B_e+8{\mu }_e)/(6B_e+12{\mu }_e)$ 。当组分 1 以椭圆粒子形式分布在背景基质中时的情况也已进行研究（Berrymnan 1980）。只要 ${\varphi }_1\ll {\varphi }_2$ ，方程（21）和（22）是准确的。

Suppose, for example, that the matrix consists of clay with a volume fraction of ${\varphi }_1=0.22$, for which ${\mu }_1=6.85$ GPa and $B_1=21$ GPa, and quartz with volume fraction of ${\varphi }_2=0.78$ with $B_2=138$ GPa and ${\mu }_2=44$ GPa. Then, Eqs. (21) and (22) yield, ${\mu }_e\approx 30.5$ GPa and $B_e\approx 33.5$ GPa. Thus, since the Poisson’s ratio is given by, $\nu =(D{B}_e-2{\mu }_e)/[D(D-1)B_e+2{\mu }_e]$, with $D=3$ being the dimensionality of the space, we obtain, $\nu \approx 0.15$. The effective Young’s modulus is then given by, $E_e=2{\mu }_e(1+\nu )\approx 70$ GPA. On the other hand, suppose that a sandstone is composed of about 24 percent quartz (component 1), the same as Fahler 154 and close to the Boise sandstone, both analyzed in Sect. 7, while the rest is made of clay (or any other compound much softer than quartz) as component 2. Then, Eqs. (21) and (22) predict that, $B_e\approx 28.7$ and ${\mu }_e\approx 10.3$, both in GPa, so that $\nu \approx 0.28$ and $E_e\approx 26$ GPa, much smaller than that of pure quartz, $E_e\approx 106$ GPa. These are, of course, approximations, but they do indicate that the Young’s modulus of the grains depends strongly on their compositions.
例如，假设矩阵由体积分数为 ${\varphi }_1=0.22$ 的粘土组成，其弹性模量为 ${\mu }_1=6.85$ GPa 和 $B_1=21$ GPa，以及体积分数为 ${\varphi }_2=0.78$ 的石英，其弹性模量为 $B_2=138$ GPa 和 ${\mu }_2=44$ GPa。那么，方程(21)和(22)给出， ${\mu }_e\approx 30.5$ GPa 和 $B_e\approx 33.5$ GPa。因此，由于泊松比由 $\nu =(D{B}_e-2{\mu }_e)/[D(D-1)B_e+2{\mu }_e]$ 给出，其中 $D=3$ 是空间的维度，我们得到 $\nu \approx 0.15$ 。有效杨氏模量随后由 $E_e=2{\mu }_e(1+\nu )\approx 70$ GPA 给出。另一方面，假设砂岩由约 24%的石英（组分 1）组成，与 Fahler 154 相同，接近博伊西砂岩，两者均在第 7 节中进行分析，其余部分由粘土（或任何比石英软得多的化合物）作为组分 2 组成。那么，方程(21)和(22)预测， $B_e\approx 28.7$ 和 ${\mu }_e\approx 10.3$ ，均为 GPa，因此 $\nu \approx 0.28$ 和 $E_e\approx 26$ GPa，远小于纯石英的 $E_e\approx 106$ GPa。当然，这些都是近似值，但它们确实表明，颗粒的杨氏模量强烈依赖于其成分。

The information on the exact composition of the solid matrix of the deforming sandstones that we analyze in the present paper is not available. Therefore, in the absence of such information that we could have used in, for example, Eqs. (21) and (22) to estimate $E_e$ for each deforming porous medium that we analyze, we utilize a single experimental data point for the permeability at a given pressure P in order to calibrate the model and estimate $E_e$. We typically took the point in the middle of the pressure range for each set of the data. The estimate is then utilized for predicting $K_e(P)$ at all other pressures.
本文中分析的变形砂岩固体基质的精确组成信息不可用。因此，在没有此类信息的情况下，我们无法使用，例如，方程（21）和（22）来估计每个分析的变形多孔介质的 $E_e$ ，我们利用一个给定的压力 P 下的渗透率实验数据点来校准模型并估计 $E_e$ 。我们通常取每组数据压力范围中间的点。然后，该估计用于预测所有其他压力下的 $K_e(P)$ 。

It is, of course, well-known that the elastic moduli of composite materials, including porous media’s solid matrix, are functions of the applied pressure P. Consider, however, the elastic moduli of quartz, which is typically a main component of sandstones. Its elastic moduli do depend on P (Kondo et al. 1981; Wang et al. 2015), but only at pressures much higher than those considered in the experiments described below. Thus, ignoring the pressure-dependence of $E_e$ is justified. For the predictions that we present below, we used a data point at a pressure in the middle of the range of the pressures at which $K_e$ had been measured.
当然，众所周知，复合材料的弹性模量，包括多孔介质的固体基质，是施加压力 P 的函数。然而，考虑石英的弹性模量，它是砂岩的主要成分。其弹性模量确实依赖于 P（Kondo 等人 1981；Wang 等人 2015），但仅在远高于以下实验中考虑的压力下。因此，忽略 $E_e$ 的压力依赖性是有道理的。对于我们下面提出的预测，我们使用了在 $K_e$ 测量的压力范围内的中间压力的数据点。

6 Computational Procedure
6 计算过程

Having developed the necessary theoretical tools for predicting the effective permeability of deforming porous media, the computational procedure is as follows.
开发了预测变形多孔介质有效渗透率所需的理论工具，计算程序如下。

• (i)（i） We begin with an initial PSD $f_0(r_0)$ of the porous medium to be deformed and estimate its initial permeability using Eqs. (18) and (19).
  我们从变形多孔介质的初始 PSD $f_0(r_0)$ 开始，并使用公式（18）和（19）估计其初始渗透率。

• (ii)（ii） For an applied hydrostatic pressure P, we construct the PSD $f_P(r_P)$ corresponding to P by selecting the pore sizes from $f_0(r_0)$, calculating their updated values using Eq. (17) and $r_P=r_0-u/2$, and repeating it for a large number of pore sizes selected from $f_0(r_0)$, in order to construct a representative $f_P(r_P)$.
  对于施加的静水压力 P，我们通过选择孔径从 $f_0(r_0)$ ，使用公式（17）和 $r_P=r_0-u/2$ 计算它们的更新值，并重复此过程，对于从 $f_0(r_0)$ 选择的大量孔径，以构建一个代表性的 $f_P(r_P)$ 。

• (iii) The resulting $f_P(r_P)$ is then utilized to first update the value of $⟨r_b^2(P)⟩=⟨r_P^2⟩$ and then is used together with $f_P(r_P)$ in Eqs. (18) and (19) to compute $K_e(P)$ at the given pressure P.
  结果的 $f_P(r_P)$ 随后被用来首先更新 $⟨r_b^2(P)⟩=⟨r_P^2⟩$ 的值，然后与 $f_P(r_P)$ 一起用于计算在给定压力 P 下的 $K_e(P)$ ，如公式（18）和（19）所示。

As pointed out earlier, if the PSD $f_0(r_0)$ can be expressed by an analytical expression, such as Eq. (20), then, so can also $f_P(r_P)$ for any P in which case the computations are very fast.
如前所述，如果 PSD $f_0(r_0)$ 可以用解析表达式表示，例如公式（20），那么对于任何 P， $f_P(r_P)$ 也可以，这样计算就非常快。

7 Results and Comparison with Experimental Data
7 结果与实验数据的比较

Let us first emphasize again that Eqs. (16)–(19) are not exact, but represent only MFAs to the problem, which we now utilize to predict the pressure-dependence of the effective permeability $K_e(P)$ of a large number of sandstones, and to compare the predictions with the experimental data. Almost all the experimental data are given by Yale (1984), although as noted below, some of them were not his measurements, but he had included them in his Doctoral Thesis for comparison and completeness. Yale (1984) did not provide the sandstones’ initial PSD and, therefore, as mentioned earlier, we used in all the cases described below the PSD presented in Fig. 2b as the initial PSD, $f_0(r_0)$, which was reported by Lindquist et al. (2000). The qualitative aspects of the PSD that we utilize are similar to those for many sandstones, namely, that the distribution is skewed; it has a maximum close to the smallest pores, and that it also has a relatively long tail. Clearly, any PSD can be used in the theoretical formulation that we have developed.
让我们首先再次强调，方程（16）-（19）并不精确，但仅代表对该问题的近似解，我们现在利用它来预测大量砂岩的有效渗透率 $K_e(P)$ 的压力依赖性，并将预测结果与实验数据进行比较。几乎所有实验数据都由耶鲁（1984 年）提供，尽管如下所述，其中一些并非他的测量结果，但他将它们包含在他的博士论文中，以进行比较和完整性。耶鲁（1984 年）没有提供砂岩的初始 PSD，因此，如前所述，我们在下面描述的所有情况下，使用图 2b 中呈现的 PSD 作为初始 PSD， $f_0(r_0)$ ，这是林奎斯特等人（2000 年）报道的。我们利用的 PSD 的定性方面与许多砂岩相似，即分布是偏斜的；它在最小孔隙附近达到最大值，并且它还有一个相对较长的尾巴。显然，任何 PSD 都可以用于我们开发的原理公式。

Let us also emphasize that since the PSD and the Poisson’s ratio are fixed in all the cases, only the initial porosity of each sample and the fitted value of the Young’s modulus are used as the parameters of the model. Despite this, as the comparison between the predictions and the experimental data indicates, the theory provides accurate predictions for almost all the cases. In the discussions that follows all the percentages and fractions that are mentioned are volumetric.
让我们也强调，由于所有情况下的 PSD 和泊松比都是固定的，因此只有每个样品的初始孔隙率和拟合的杨氏模量被用作模型的参数。尽管如此，正如预测值与实验数据的比较所表明的，该理论几乎对所有情况都提供了准确的预测。在随后的讨论中，所有提到的百分比和分数都是体积百分比。

7.1 Fontainebleau Sandstone
7.1 丰坦布勒砂岩

Before presenting the predictions for the sandstones that Yale (1984) experimented on, we present the results for a Fontainebleau sandstone, since its PSD was reported by Lindquist et al. (2000), while the data for its pressure-dependent $K_e(P)$ were reported by Song and Renner (2008). The initial porosity of the samples was between 0.025 and 0.09 (several samples were experimented on). We found the best estimate for the Young’s modulus of the sandstone that provides accurate predictions for the permeability to be, $E_e\approx 30$ GPa. Figure 5 compares the predictions with the experimental data.
在展示耶鲁（1984）实验的砂岩预测之前，我们首先展示了一种枫丹白露砂岩的结果，因为其粒度分布（PSD）由林奎斯特等人（2000）报道，而其压力依赖性数据由宋和雷纳（2008）报道。样品的初始孔隙率在 0.025 到 0.09 之间（对多个样品进行了实验）。我们发现，对于提供准确渗透率预测的砂岩，其杨氏模量的最佳估计值为， $E_e\approx 30$ GPa。图 5 比较了预测值与实验数据。

Fig. 5
图 5

Comparison of the predicted permeabilities with the experimental data for the Fontainebleau sandstone. The estimate value of the Young’s modulus $E_e$ of the grains is $\approx 30$ GPa
比较预测的渗透率与 Fontainebleau 砂岩的实验数据。颗粒的杨氏模量 $E_e$ 的估计值为 $\approx 30$ GPa

One may reasonably argue that the predictions are not as accurate as one would expect, since the “universal” PSD that we used for all the cases was taken from the data for this Fontainebleau sandstone. Indeed, in this case the model is actually the least successful (see below), since normalized permeability is under- or over-predicted for small or large P, with the overall slope being about half of the experimental data. If, as a reviewer pointed out, we simply model $K_e(P)/K_e(0)=1$, we will have an error of about 15 percent over the wide range of pressures used in the experiments, which should be compared with the maximum error of about 4.4 percent, produced by the model, which is definitely an improvement but, perhaps, one might expect a better performance by the model.
可以合理地认为，预测结果并不像预期的那样准确，因为我们用于所有情况的“通用”PSD 是从这个 Fontainebleau 砂岩的数据中提取的。实际上，在这种情况下，模型实际上是最不成功的（见下文），因为对于小或大的 P，归一化渗透率被低估或高估，整体斜率大约是实验数据的一半。如果，正如一位审稿人指出的那样，我们仅仅对 $K_e(P)/K_e(0)=1$ 进行建模，那么在实验中使用的广泛压力范围内，我们将有大约 15%的误差，这应该与模型产生的最大误差约 4.4%进行比较，这确实是一个改进，但也许，人们可能期望模型有更好的表现。

It is, however, noteworthy to point out that the data are considerably scattered. Moreover, the normalized permeability at a pressure of about 180 MPa (the second data point from the left) is larger than 1, hence, indicating that, during the experiments, something might have happened to the sample that the theory cannot account for. Note also that the data vary in a narrow range of (0.85,1) and, therefore, the apparent disagreement between the predictions and the data is a bit superficial. In fact, as mentioned earlier, the maximum error of the predictions is only 4.4 percent, while the average error is only 2 percent.
然而，值得注意的是，数据分布相当分散。此外，在约 180 MPa 的压力下（从左数第二个数据点），归一化渗透率大于 1，因此，在实验过程中，可能发生了理论无法解释的样本变化。请注意，数据在(0.85,1)的狭窄范围内变化，因此，预测值与数据之间的明显不一致只是表面上的。事实上，如前所述，预测的最大误差仅为 4.4%，而平均误差仅为 2%。

Let us also point out that Fredrich et al. (1993) also reported experimental data for the PSD and pressure-dependence of the permeability of a Fontainebleau sandstone. Their PSD has striking similarity with the distribution that Eq. (20) produces for $r_0=1$. However, their data for $K_e(P)$ have some peculiar features. In particular (see their Fig. 4), $K_e(P)$ sharply drops when what is referred to as the “effective pressure” is increased from zero to only 4 MPa. It then stays essentially constant for 4 MPa $<P<$ 40 MPa, and then it increases for $P>40$ MPa. Fredrich et al. (1993) remarked that their data have considerable scattering due to inelastic deformations; that some data may be “artifacts” at the higher pressures due to errors in the opening and closing valves, and that the core sample that they experimented on split in half when they removed it from the experimental setup. Thus, we did not try to predict their data.
让我们也指出，Fredrich 等人（1993 年）也报道了关于 Fontainebleau 砂岩的 PSD 和渗透率压力依赖性的实验数据。他们的 PSD 与公式（20）产生的分布有惊人的相似性。然而，他们关于 $K_e(P)$ 的数据有一些奇特的特征。特别是（参见他们的图 4），当所谓的“有效压力”从零增加到仅 4 MPa 时， $K_e(P)$ 会急剧下降。然后它对于 4 MPa $<P<$ 40 MPa 基本保持不变，然后它对于 $P>40$ MPa 开始增加。Fredrich 等人（1993 年）指出，他们的数据有相当大的分散性，这是由于非弹性变形造成的；在较高的压力下，一些数据可能是由于开启和关闭阀门错误而造成的“伪影”，并且他们在从实验装置中取出样品时，样品被分成两半。因此，我们没有尝试预测他们的数据。

Yale (1984) stated that in all cases that he experimented on the pore pressure $P_p$ was constant. Thus, in what follows the pressure P may be replaced by $P-P_p$.
Yale（1984 年）指出，在他所进行的所有实验中，孔隙压力 $P_p$ 都是恒定的。因此，在下面的讨论中，压力 P 可以被替换为 $P-P_p$ 。

7.2 Beaver River Sandstone
7.2 比弗河砂岩

Fig. 6
图 6

Comparison of the predicted permeabilities with the experimental data for the Beaver sandstone. The estimated value of the Young’s modulus $E_e$ of the grains is 2.7 GPa
预测渗透率与实验数据对比，比弗砂岩的颗粒的杨氏模量估算值为 2.7 GPa

The Beaver River sandstone (BRS) is a formation on the west side of the Athabasca River near Mildred Lake and the Beaver River (in Alberta, Canada) and has been identified as quartzite (Kristensen et al. 2015) with fine- to medium-size and well-sorted grains, 78 percent of which was quartz. Its pore space was cemented by 16 percent quartz overgrowth and also contained clay. Its initial porosity (before deformation) was ${\varphi }_0\approx 0.076$. Figure 6 compares the predicted permeabilities, normalized by the initial permeability of the sandstone before deformation (as also presented by Yale (1984)) as a function of the applied pressure, with the experimental data of Yale (1984). The agreement between the two is excellent. Because the sandstone is mostly quartz, its porosity and, thus, PSD do not change much over the range of the applied pressure. This is confirmed by the inset of Fig. 6 that shows that the porosity is reduced by only 5 percent of its initial value, as well as Fig. 7 that presents the PSD of the sandstone at higher pressures, indicating only small changes.
比弗河砂岩（BRS）是加拿大艾伯塔省米尔德里德湖和比弗河（阿萨巴斯卡河西岸）附近的一种地层，已被确认为石英岩（Kristensen 等人，2015 年），具有细到中等的粒度，其中 78%为石英。其孔隙空间被 16%的石英过生长水泥化，还含有粘土。其初始孔隙率（变形前）为 ${\varphi }_0\approx 0.076$ 。图 6 比较了预测的渗透率（以变形前砂岩的初始渗透率归一化，如耶鲁（1984 年）所展示）作为施加压力的函数，与耶鲁（1984 年）的实验数据。两者之间的一致性非常好。由于砂岩主要是石英，其孔隙率和因此 PSD 在施加压力的范围内变化不大。图 6 的插入图证实了这一点，显示孔隙率仅降低了其初始值的 5%，以及图 7 展示了在更高压力下砂岩的 PSD，表明只有微小变化。

Fig. 7
图 7

Evolution of the pore-size distribution of the Beaver sandstone as a result of applying the external hydrostatic pressure P to the sandstone
外部静水压力 P 作用于砂岩后，比埃拉砂岩孔隙尺寸分布的变化

7.3 Berea Sandstones
7.3 贝雷砂岩

Yale (1984) presented experimental data for two Berea sandstone. One was Berea 100H (with H implying that the bedding was horizontal in the experiments) with a sublitharenite environment—one in which the sandstone is characterized by the presence of less than 15 percent mud matrix—with 5–25% of the grains being rock fragments, more than the feldspar content. The sandstone consisted of 53% quartz and had fine to very fine, well-sorted grains with an initial porosity of 0.165. Its cement contained 11% quartz overgrowth, as well as clay. Figure 8a compares the predicted permeabilities with the experimental data; the agreement is excellent.
耶鲁（1984）提供了两种贝雷亚砂岩的实验数据。一种是贝雷亚 100H（H 表示在实验中层理是水平的）具有次长石环境——在这种环境中，砂岩以少于 15%的泥质基质为特征，5-25%的颗粒是岩石碎片，多于长石含量。砂岩由 53%的石英组成，具有细到非常细、分选良好的颗粒，初始孔隙率为 0.165。其胶结物含有 11%的石英生长物以及粘土。图 8a 比较了预测的渗透率与实验数据；一致性非常好。

Fig. 8
图 8

Comparison of the predicted permeabilities with the experimental data for two Berea sandstones. a Berea 100H with fitted value of the Young’s modulus $E_e\approx 25$ GPa, and b Berea 500 with $E_e\approx 13$ GPa
比较预测渗透率与两种贝雷阿砂岩的实验数据。a 贝雷阿 100H，其杨氏模量拟合值为 $E_e\approx 25$ GPa，b 贝雷阿 500，其值为 $E_e\approx 13$ GPa。

Fig. 9
图 9

Comparison of the predicted permeabilities with the experimental data for Boise sandstone with the fitted value of the Young’s modulus $E_e\approx 38.5$ GPa
预测渗透率与实验数据对比，Boise 砂岩，拟合的杨氏模量为 $E_e\approx 38.5$ GPa

The second Berea sandstone that Yale (1984) experimented on was Berea 500, a quartzenite-type porous medium composed of up to 90 percent detrital quartz, with limited amounts of other framework grains, such as feldspar and lithic fragments. Such sandstone can have higher-than-average amounts of resistant grains, such as chert and minerals. Sixty six percent of Berea 500 was quartz. Its initial porosity was ${\varphi }_0\approx 0.2$, while its cement consisted of 5 percent quartz overgrowth, 8 percent Fe oxide, and 1 percent clay, with the rest being other types of materials. Figure 8b presents a comparison of the pressure-dependence of the permeability with the experimental data, indicating excellent agreement. In both sandstones, the porosity was reduced by only 5-6 percent of its initial value over the entire range of pressure and, therefore, the change in the PSDs was small.
耶鲁（1984）实验的第二种 Berea 砂岩是 Berea 500，这是一种由高达 90%的碎屑石英组成的石英砂岩型多孔介质，含有有限的其他骨架颗粒，如长石和岩屑碎片。这种砂岩可以含有高于平均量的抗磨颗粒，如燧石和矿物。Berea 500 中有 66%是石英。其初始孔隙率为 ${\varphi }_0\approx 0.2$ ，而其水泥由 5%石英生长、8%氧化铁和 1%粘土组成，其余为其他类型的材料。图 8b 展示了渗透率压力依赖性与实验数据的对比，表明吻合良好。在这两种砂岩中，孔隙率在整个压力范围内仅降低了其初始值的 5-6%，因此 PSD 的变化很小。

7.4 Boise Sandstone
7.4 博伊西砂岩

The Boise sandstone used in the experiments by Yale (1984) was of arkosic arenite, or arkose type, a detrital sedimentary rock that contains at least 25% feldspar, which is why it is sometimes referred to loosely as feldspathic sandstone. The sandstone was fine to medium grained, very well sorted, with initial porosity of about 0.26 and minor carbonate-clay cement. Its grains consisted of 28 percent quartz and 44 percent feldspar. Figure 9 compares the predicted permeabilities w the estimated $E_e\approx 38.5$ GPa is close to what the SCA, Eqs. (21) and (22), predict, $E_e\approx 26$ GPa. is close to what the SCA, Eq.s (21) and (22), predict, $E_e\approx 26$ GPa.
实验中使用的耶鲁大学（1984 年）的博伊西砂岩为长石砂岩，或称为长石质砂岩，是一种含有至少 25%长石的碎屑沉积岩，因此有时被松散地称为长石砂岩。该砂岩为细至中等粒度，非常分选，初始孔隙率约为 0.26，含有少量碳酸盐-粘土胶结物。其颗粒由 28%石英和 44%长石组成。图 9 比较了预测的渗透率与 SCA（方程式（21）和（22））预测的值，在 0# $E_e\approx 38.5$ GPa 时接近，在 $E_e\approx 26$ GPa 时接近，在 $E_e\approx 26$ GPa 时接近。

7.5 Cambrian Sandstone
7.5 寒武纪砂岩

Fig. 10
图 10

Comparison of the predicted permeabilities with the experimental data for three Cambrian sandstones with the fitted value of the Young’s modulus being a $E_e\approx 10$; b 12, and c 2, all in GPa
将预测的渗透率与三个寒武纪砂岩的实验数据进行比较，拟合的杨氏模量为 a $E_e\approx 10$ ; b 12，和 c 2，均为 GPa

Chierici et al. (1967) presented experimental data for the pressure-dependence of the permeability of three Cambrian sandstones. These are low-porosity sandstones from the Cambrian era that consist of sand-size quartz grains held together by quartz cement. The three were referred to as Cambrian 6, Cambrian 14 and Cambrian 16 by Yale (1984) and had initial porosities of 0.08, 0.11, and 0.13, respectively. Figure 10a–c presents comparison of the predicted pressure-dependence of the effective permeability with the experimental data. In all cases, the agreement between the predictions and the data is good, with the largest difference being about 12 percent at 45 MPa, applied to Cambrian 16. Note that the fact that in the cases of Cambrian 6 and Cambrian 14 the final porosities of the sandstones at the highest pressure applied were about 95 percent of their initial values indicates the rigidity of their structure. In addition, the lower value of the Young’s modulus $E_e$ for the Cambrian 16 is consistent with a larger reduction in its initial porosity.
Chierici 等人（1967 年）提供了三个寒武纪砂岩的渗透率压力依赖性的实验数据。这些是寒武纪时代低孔隙率的砂岩，由砂粒大小的石英颗粒组成，由石英胶结剂粘合在一起。这三个分别被称为寒武纪 6 号、寒武纪 14 号和寒武纪 16 号，由耶鲁（1984 年）命名，初始孔隙率分别为 0.08、0.11 和 0.13。图 10a-c 展示了预测的有效渗透率压力依赖性与实验数据的比较。在所有情况下，预测值与数据之间的吻合度良好，最大差异约为 12%，在 45 MPa 下应用于寒武纪 16 号。请注意，在寒武纪 6 号和寒武纪 14 号的情况下，在施加最高压力时砂岩的最终孔隙率约为初始值的 95%，这表明其结构的刚性。此外，寒武纪 16 号杨氏模量 $E_e$ 的较低值与其初始孔隙率的大幅减少一致。

7.6 Fahler Sandstones
7.6 法勒砂岩

Fig. 11
图 11

Comparison of the predicted permeabilities with the experimental data for the four Fahler sandstones with the fitted value of the Young’s modulus being a $E_e\approx 0.12$; b 0.15; c 0.11, and d 0.17, all in GPa
预测渗透率与四种 Fahler 砂岩的实验数据的比较，拟合的杨氏模量为 a $E_e\approx 0.12$ ; b 0.15; c 0.11，d 0.17，均为 GPa

Yale (1984) presented experimental data for pressure-dependence of the effective permeabilities of four Fahler sandstones, which he referred to them as Falher 142, Fahler 154, Fahler 162, and Fahler 189. Of the four, Fahler 142 was of quartzarenite type, whereas the other three were sublitharenite sandstones. Moreover, the initial porosity of Fahler 142 was ${\varphi }_0\approx 0.08$. It was fine grained, very well sorted, with quartz outgrowth, and clay and carbonate cement. It consisted of 35 percent quartz, 8 percent chert, and 3 percent feldspar, with the rest being various other types of rock materials. Its cement contained 23 percent carbonate. Fahler 154 with an initial porosity of 0.044 was very fine to fine grained and very well sorted, 24 percent of which was quartz, 8 percent chert, 4 percent lithics, and 2 percent feldspar, with the rest being other rock materials. Its cement contained chalcedony (a cryptocrystalline form of silica), Fe oxide and carbonate. Likewise, Fahler 162 was a sandstone with an initial porosity of 0.03, fine to medium grained, consisting of 46 percent quartz, 8 percent various lithics, and 6 percent chert, with the rest being other types of rock materials. Its cement consisted of 25 percent quartz overgrowth, 8 percent Fe oxide, and 8 percent clay. Finally, Fahler 189 had an initial porosity of 0.02, medium grained, and contained 27 percent quartz, 27 percent chert, 11 percent various lithics, and 3 percent feldspar. Its cement consisted of 13 percent quartz overgrowth, 9 percent carbonate, 4 percent chalcedony, and 2 percent clay.
耶鲁（1984 年）提供了四种法勒砂岩的有效渗透率随压力变化的实验数据，他将它们称为法勒 142 号、法勒 154 号、法勒 162 号和法勒 189 号。在这四种中，法勒 142 号是石英砂岩类型，而其他三种是次长石砂岩。此外，法勒 142 号的初始孔隙率为 ${\varphi }_0\approx 0.08$ 。它是细粒的，非常分选，有石英突出，以及粘土和碳酸盐胶结。它由 35%石英、8%燧石和 3%长石组成，其余为各种其他类型的岩石材料。其胶结物中含有 23%碳酸盐。初始孔隙率为 0.044 的法勒 154 号非常细到细粒，非常分选，其中 24%为石英、8%燧石、4%岩屑和 2%长石，其余为其他岩石材料。其胶结物中含有玉髓（石英的隐晶质形式）、氧化铁和碳酸盐。同样，初始孔隙率为 0.03 的法勒 162 号是一种砂岩，细到中粒，由 46%石英、8%各种岩屑和 6%燧石组成，其余为其他类型的岩石材料。 其水泥由 25%石英过生长、8%氧化铁和 8%粘土组成。最后，Fahler 189 的初始孔隙率为 0.02，中等粒度，含有 27%石英、27%燧石、11%各种岩屑和 3%长石。其水泥由 13%石英过生长、9%碳酸盐、4%玉髓和 2%粘土组成。

Figure 11a–d presents the predictions and compares them with the experimental data. We first note the consistency between the estimated values of $E_e$ for the four sandstones, which indicates the internal consistency of the theoretical model. As Figure 11 indicate, in all cases the agreement between the predictions and the data is excellent for pressures as high as 25 MPa, but they deviate from the data at higher pressures. We will come back to this point in Sect. 8.
图 11a-d 展示了预测结果，并将其与实验数据进行比较。我们首先注意到对于四种砂岩， $E_e$ 的估计值之间的一致性，这表明了理论模型的内部一致性。如图 11 所示，在所有情况下，预测值与数据在高达 25 MPa 的压力下都表现出优异的一致性，但在更高的压力下与数据有所偏差。我们将在第 8 节中回到这一点。

Fig. 12
图 12

Comparison of the predicted permeabilities with the experimental data for the Indiana DH sandstone with the fitted value of the Young’s modulus being $E_e\approx 30$ GPa
将预测的渗透率与印第安纳 DH 砂岩的实验数据进行比较，拟合的杨氏模量为 $E_e\approx 30$ GPa

7.7 Indiana Dark Sandstone
7.7 印第安纳深色砂岩

The next sandstone whose pressure-dependent permeability we predicted is the Indiana Dark sandstone, referred to as the Indiana DH, with DH indicating that the sample was taken after drilling horizontally. The sandstone was of subarkose type, one in which feldspar sand grains exceed rock fragments, but make up 5 to 15 percent of the rock. Its initial porosity was relatively high, 0.27, with its major components being 57 percent quartz and 7 percent feldspar. Its cement contained clay, as well as 22 percent hematite, Fe${}_2$O${}_3$. Figure 12 compares the predictions with the experimental data of Yale (1984); the agreement is excellent. Note that since the initial porosity of the sandstone was high and it reduced by only 4 percent even at the highest pressure, then, consistent with our arguments about the Fahler sandstone, the porous medium remained well connected, precisely in the range of porosity in which the EMA is highly accurate.
下一个我们预测其压力依赖性渗透率的砂岩是印第安纳深色砂岩，称为印第安纳 DH，其中 DH 表示该样品是在水平钻探后采集的。这种砂岩属于亚长石砂岩类型，其中长石砂粒超过岩石碎片，但占岩石的 5 至 15%。其初始孔隙率相对较高，为 0.27，其主要成分是 57%的石英和 7%的长石。其胶结物含有粘土，以及 22%的赤铁矿，Fe ${}_2$ O ${}_3$ 。图 12 比较了预测值与耶鲁（1984 年）的实验数据；一致性非常好。请注意，由于砂岩的初始孔隙率较高，即使在最高压力下也仅减少了 4%，因此，与我们对法勒砂岩的论点一致，多孔介质保持了良好的连通性，正好处于 EMA 高度准确的孔隙率范围内。

7.8 Massillon Dark Sandstone
7.8 马萨隆深色砂岩

Fig. 13
图 13

Comparison of the predicted permeabilities with the experimental data for the Massillon DH sandstone with the fitted value of the Young’s modulus being $E_e\approx 9.5$ GPa
预测渗透率与实验数据对比，Massillon DH 砂岩（来自俄亥俄州 Stark 县 Massillon，耶鲁 1984 年命名）的杨氏模量拟合值为 0# $E_e\approx 9.5$ GPa

The sandstone, referred to as Massillon DH (from Massillon, Stark County, Ohio) by Yale (1984), was of quatzarenite type with an initial porosity of 0.161, and medium-size and well-sorted grains. Sixty-one percent of it was quartz, with feldspar, chert, and lithics each contributing 1 percent, and the rest being other types of rock material. Its cement contained Fe oxide at 15 percent and clay at 5 percent. Figure 13 compares the predictions with the experimental data of Yale (1984). The largest difference between the two sets is about 6 percent at a pressure of 40 MPa.
该砂岩被称为 Massillon DH（来自俄亥俄州 Stark 县 Massillon，耶鲁 1984 年命名），为石英砂岩类型，初始孔隙率为 0.161，粒度中等且分选良好。其中 61%为石英，长石、燧石和岩屑各占 1%，其余为其他类型的岩石材料。其胶结物中含有 15%的氧化铁和 5%的粘土。图 13 比较了预测值与耶鲁（1984 年）的实验数据。两组数据之间的最大差异约为 6%，发生在 40 MPa 的压力下。

7.9 Miocene Sandstone
790 万年前砂岩

Fig. 14
图 14

Comparison of the predicted permeabilities with the experimental data for the Miocene 7 sandstone with the fitted value of the Young’s modulus being $E_e\approx 5$ GPa
与拟合的杨氏模量为 $E_e\approx 5$ GPa 的渐新世 7 号砂岩的预测渗透率与实验数据的比较

Chierici et al. (1967) reported experimental data for the pressure-dependence of Miocene sandstone, a low-porosity rock that is of the feldspathic arenite type. Its initial porosity was 0.083. The roundness and sorting of the grains of such sandstones are typically high, implying the existence of long flow and transport distances (Saitoh and Masuda 2004). Figure 14 presents the comparison between the predictions for the pressure-dependent permeability and the experimental data. The agreement is excellent. The existence of well-connected and long transport and flow paths practically guarantees that the predictions would be accurate, because it is precisely under such conditions that the EMA is accurate.
Chierici 等人（1967 年）报道了米奥辛砂岩的压力依赖性实验数据，这是一种低孔隙率的岩屑长石砂岩。其初始孔隙率为 0.083。这种砂岩的颗粒圆度和分选度通常很高，这意味着存在较长的流动和传输距离（Saitoh 和 Masuda 2004）。图 14 展示了压力依赖性渗透率预测与实验数据的比较。一致性非常好。存在良好连接的长期传输和流动路径实际上保证了预测的准确性，因为正是在这种条件下 EMA 才是准确的。

7.10 Pliocene Sandstone
更新世砂岩

Fig. 15
图 15

Comparison of the predicted permeabilities with the experimental data for the Pliocene 35 sandstone with the fitted value of the Young’s modulus being $E_e\approx 4$ GPa
预测渗透率与实验数据对比，Pliocene 35 砂岩，拟合的杨氏模量为 $E_e\approx 4$ GPa

Chierici et al. (1967) also reported experimental data for the pressure-dependence of the permeability of a Pliocene sandstone, which Yale (1984) referred to it as Pliocene 35. Similar to Miocene sandstones, Pliocene sandstones also have round grains. The initial porosity of the sample was 0.2. Figure 15 compares the predictions with the data. The agreement between the two is excellent.
Chierici 等人（1967 年）也报道了关于上新世砂岩渗透率压力依赖性的实验数据，耶鲁（1984 年）将其称为 Pliocene 35。与中新世砂岩类似，上新世砂岩也具有圆形颗粒。样品的初始孔隙率为 0.2。图 15 比较了预测值与数据。两者之间的一致性非常好。

7.11 Tensleep Sandstone
7.11 tensleep 砂岩

Fatt (1957) reported on his measurements of the pressure-dependence of the permeability of Tensleep sandstone. The porous medium represents a geological formation from the entire Pennsylvanian sequence in central and northern Wyoming in the very early Permian age (Branson and Branson 1941). Such rocks are predominantly crossbedded sandstone and have thin limestone and dolomite beds (Kerr et al. 1986). The initial porosity of the sample was 0.146. In Fig. 16, we compare the predictions with the experimental data of Fatt (1957), also reported by Yale (1984). The agreement is excellent over much of the range of the applied pressure. The largest difference, at the highest pressure, is about 10 percent.
Fatt（1957 年）报告了他对 Tensleep 砂岩渗透率压力依赖性的测量。多孔介质代表的是来自中西部和北部怀俄明州整个宾夕法尼亚系地层，在早二叠纪非常早期的地质构造（Branson 和 Branson 1941）。这类岩石主要是交错层砂岩，并含有薄层的石灰岩和白云岩（Kerr 等人 1986）。样品的初始孔隙率为 0.146。在图 16 中，我们比较了预测值与 Fatt（1957 年）的实验数据，以及耶鲁（1984 年）的报告。在应用压力的大部分范围内，一致性非常好。在最高压力下，最大差异约为 10%。

Fig. 16
图 16

Comparison of the predicted permeabilities with the experimental data for the Tensleep 35 sandstone with the fitted value of the Young’s modulus being $E_e\approx 2.6$ GPa
与 Tensleep 35 砂岩的实验数据预测渗透率与拟合的杨氏模量值 $E_e\approx 2.6$ GPa 的比较

Fig. 17
图 17

Comparison of the predicted permeabilities with the experimental data for the Tertiary 807 sandstone with the fitted value of the Young’s modulus being $E_e\approx 6.4$ GPa
预测渗透率与实验数据对比，Tertiary 807 砂岩，拟合的杨氏模量为 $E_e\approx 6.4$ GPa

Fig. 18
图 18

Comparison of the predicted permeabilities with the experimental data for the Torpedo sandstone with the fitted value of the Young’s modulus being $E_e\approx 3$ GPa
预测渗透率与实验数据的对比，对于具有拟合杨氏模量 $E_e\approx 3$ GPa 的鱼雷砂岩

7.12 Gulf Coast Sandstone
7.12 加尔维斯顿湾砂岩

The experimental data for this sandstone were reported by Yale (1984), who referred to the rock as Tertiary 807. In general, tertiary rocks are those that were formed during part of the Cenozoic era, covering the Paleogene and Neogene periods. The sample with which the experiments were carried out was of the subarkose type, with fine, well-sorted grains and high intergranular porosity. It contained 52 percent quartz, 9 percent feldspar, 5 percent chert, and 3 percent lithics with an initial porosity of 0.22. Figure 17 compares the theoretical predictions with the experimental data. Once again, over much of the pressure range the agreement between the predictions and the data is excellent. The theory predicts slightly higher permeabilities at the two highest pressures, with the largest deviation from the data being, however, about 5 percent at 40 MPa.
该砂岩的实验数据由耶鲁（1984 年）报道，他将该岩石称为第三纪 807 号。一般来说，第三纪岩石是指在古近纪和中新世期间形成的岩石，涵盖了古近纪和中新世。进行实验的样品为亚砂屑岩类型，具有细粒、分选良好的颗粒和高孔隙率。它含有 52%的石英、9%的长石、5%的燧石和 3%的岩屑，初始孔隙率为 0.22。图 17 比较了理论预测与实验数据。再次证明，在大部分压力范围内，预测值与数据之间的吻合度非常好。理论预测在两个最高压力下渗透率略高，但与数据相比，最大偏差约为 5%，在 40 MPa 时。

7.13 Torpedo Sandstone
7.13 鱼雷砂岩

Dobrynin (1962) reported permeability data for the Torpedo sandstone from Kansas. The initial porosity of the sample was 0.202, and it contained about 5 percent clay minerals that consisted mostly of kaolinite and chlorite, distributed evenly throughout the sample. As Figure 18 indicates, except at 45 MPa where the predicted permeability is larger than the measured value by 5 percent, the agreement between the predictions and the experimental data is excellent.
Dobrynin（1962）报道了堪萨斯州 Torpedo 砂岩的渗透率数据。样品的初始孔隙率为 0.202，含有约 5%的粘土矿物，主要由高岭石和绿泥石组成，分布在整个样品中。如图 18 所示，除了在 45 MPa 处预测的渗透率比测量值大 5%外，预测值与实验数据之间的一致性非常好。

7.14 Triassic Sandstones
三叠纪砂岩

Yale (1984) reported measurements of the pressure-dependence of the permeability of five Triassic sandstones. Such rocks were formed in the Triassic period, between 200 and 251 million years ago. The morphology of such sandstones varies greatly, from very fine- to very coarse-grained. They represent porous formations with low- or ultra-low permeability, but they often contain both tectonic and diagenetic fractures that provide flow paths. The five sandstones studied by Yale (1984) were referred to as Triassic 26, 27, 34, 38, and 41 with initial porosities that were, respectively, 0.18, 0.18, 0.2, 0.2, and 0.21. Figure 19a–e compare the predictions of the permeabilities with the experimental data. Except for Triassic 27 sandstone, the agreement between the predictions and the data is uniformly excellent. Even in the case of Triassic 27, the maximum difference between the predictions and the data at high pressures is only about 5 percent.
耶鲁（1984 年）报道了对五种三叠纪砂岩渗透率压力依赖性的测量。这些岩石形成于 2 亿至 2.51 亿年前的三叠纪。这种砂岩的形态变化很大，从非常细粒到非常粗粒。它们代表具有低或超低渗透率的孔隙形成物，但它们通常含有构造和成岩裂缝，提供了流动路径。耶鲁（1984 年）研究的五种砂岩分别被称为三叠纪 26 号、27 号、34 号、38 号和 41 号，其初始孔隙率分别为 0.18、0.18、0.2、0.2 和 0.21。图 19a-e 比较了渗透率的预测值与实验数据。除了三叠纪 27 号砂岩外，预测值与数据之间的吻合度普遍非常好。即使在三叠纪 27 号砂岩的情况下，高压下预测值与数据之间的最大差异也只有大约 5%。

Fig. 19
图 19

Comparison of the predicted permeabilities with the experimental data for the Triassic sandstone with the fitted value of the Young’s modulus $E_e$ being a 8; b 6.5; c 83; d 40, and e 2.8, all in GPa
将预测的渗透率与三叠纪砂岩的实验数据进行比较，其中拟合的杨氏模量为 $E_e$ ，分别为 8；b 6.5；c 83；d 40，以及 e 2.8，单位均为 GPa

8 Discussion
第 8 节 讨论

Let us first point out that in a previous paper (Richesson and Sahimi 2019), we used the radius of contact $R_c$, given by Eq. (16) (originally presented by Yale (1984), without derivation) in order to develop an expression for the PSD of a deforming porous medium. Within the MFA that we have developed, ${\ell }_p(P)$, the pore length at pressure P, is given by (Yale 1984)
首先，我们指出，在先前的一篇论文（Richesson 和 Sahimi 2019）中，我们使用了接触半径 $R_c$ ，由公式（16）给出（最初由耶鲁（1984）提出，未进行推导），以便开发一个变形多孔介质 PSD 的表达式。在我们开发的 MFA 中 ${\ell }_p(P)$ ，在压力 P 下的孔隙长度由（耶鲁 1984）给出

$$\begin{array}{r}{\ell }_p(P)={\ell }_0-R_c(P)={\ell }_0[1-\frac{R_c(P)}{{\ell }_0}],\end{array}$$

(23)

where ${\ell }_0$ is the length of the pore before deformation. If $r_0/{\ell }_0$ is the aspect ratio of a pore before it is deformed, then, within the MFA the pore size at pressure P is given by
其中 ${\ell }_0$ 是变形前的孔隙长度。如果 $r_0/{\ell }_0$ 是变形前的孔隙长宽比，那么，在 MFA 中，在压力 P 下的孔隙尺寸由

$$\begin{array}{r}{r}_P(P)={\ell }_p(P)\left(\frac{r_0}{{\ell }_0}\right).\end{array}$$

(24)

Thus, if a hydrostatic pressure P is applied to a porous medium, the change in any pore size can be computed by Eq. (24), implying that the initial PSD can be updated. However, although as we demonstrated previously (Richesson and Sahimi 2019), we obtained excellent agreement between the predicted permeabilities and the experimental data for five sandstones, when we used Eq. (24) for updating the PSDs of the sandstones considered in this paper, good agreement between the theoretical predictions and the data could often be obtained only when the Young’s modulus $E_e$ of the grains or the solid matrix was unphysically very high, ranging from hundreds of GPa to even thousands. Thus, we believe that only when the change in the PSD is determined through the quantity u, given by Eq. (17), can one expect physically acceptable fitted values of $E_e$ and good agreement between the predictions and the experimental data.
因此，如果对一个多孔介质施加静水压力 P，任何孔隙尺寸的变化可以通过公式（24）计算得出，这意味着初始的粒度分布可以更新。然而，尽管我们之前已经证明（Richesson 和 Sahimi 2019），当我们使用公式（24）更新本文中考虑的砂岩的粒度分布时，预测的渗透率与五块砂岩的实验数据之间取得了良好的吻合，但当颗粒或固体基质的杨氏模量 $E_e$ 非常高，从数百 GPa 甚至到数千 GPa 时，理论上预测与数据之间往往只能获得良好的吻合。因此，我们相信，只有当通过公式（17）给出的量 u 确定 PSD 的变化时，才能期望得到物理上可接受的拟合值 $E_e$ ，并且预测与实验数据之间有良好的吻合。

Several other aspects of our proposed model deserve discussions, which we now present.
我们提出的模型还有其他几个方面值得讨论，我们现在来介绍。

8.1 The Fitted Values of the Young’s Modulus
8.1 杨氏模量的拟合值

As discussed in detail earlier, if for each sandstone that we examined the composition of the grains are available, then, there are a variety of theoretical methods by which their effective Young’s modulus can be estimated. In the absence of such information, we used a single point to estimate the modulus, which is similar to calibrating an experimental system. Table 1 presented the fitted values of the Young’s moduli for the sandstones considered in this paper.
如前所述，如果对于我们所检查的每一块砂岩，其颗粒成分都可用，那么可以通过多种理论方法来估计其有效杨氏模量。在没有此类信息的情况下，我们使用一个点来估计模量，这类似于校准一个实验系统。表 1 展示了本文所考虑砂岩的杨氏模量的拟合值。

As Table 1 indicates, in many cases the fitted values of the Young’s modulus are smaller than what one would expect, particularly for sandstones in which quartz is a major component. The reason is, however, clear: we fit the permeability at one point for a sandstone as a whole in order to estimate the modulus for its grains or the solid matrix. Clearly, the datum represents and is influenced by, a variety of morphological factors and, therefore, the estimate of the modulus should be smaller than the actual value because, for example, the datum is influenced by the porosity, whereas the grain themselves are solid particles. We shall comeback to this point in Sect. 8.6
如表 1 所示，在许多情况下，拟合得到的杨氏模量值小于预期，尤其是对于石英为主要成分的砂岩。然而，原因却是明显的：我们为了估计砂岩颗粒或固体基质的模量，将整个砂岩的渗透率拟合在一个点上。显然，这个数据代表并受到多种形态因素的影响，因此，模量的估计值应该小于实际值，因为例如，数据受到孔隙率的影响，而颗粒本身是固体颗粒。我们将在第 8.6 节回到这一点。

Table 1 Fitted values of the Young’s modulus $E_e$ of the sandstones’ grains
表 1 砂岩颗粒的杨氏模量拟合值 $E_e$

8.2 Limits of Validity of the EMA
8.2 EMA 有效性的限制

An important question is the range of the validity of the EMA, as well as the MFA that we developed for the deformation. Comprehensive discussions of the strengths and shortcomings of the EMA are provided by Sahimi (2003) and Hunt and Sahimi (2017). Koplik (1981), Adler and Berkowitz (2000), and others (see Sahimi 2003) studied the limit of the accuracy of the EMA. Generally speaking, the EMA is (i) accurate if a porous medium is not near its percolation threshold, i.e., the critical porosity at which the permeability and electrical conductivity vanish; (ii) more accurate for two-dimensional (2D) media than for 3D if they are close to the percolation threshold, and (iii) not very accurate for 3D porous media in what is called the critical region. In random media, the critical region is defined roughly by (Sahimi 1994), $\varphi -{\varphi }_c\le 1/Z$, where Z is the mean pore connectivity, and ${\varphi }_c$ is the critical porosity. (iv) If there are extended correlations between the pores’ sizes, then the EMA is less accurate than in completely random porous media, although Mukhopadhyay and Sahimi (2000) suggested ways of taking into account the effect of such correlations. The extensive comparison between the theoretical predictions and the experimental data presented earlier is consistent with this picture, namely, if the porosity of a porous medium is not extremely low, to the extent that it is barely connected, the MFA developed in this paper together with the EMA provide accurate predictions for the effective permeability of porous media.
一个重要的问题是 EMA 的有效范围，以及我们为变形开发的 MFA。Sahimi（2003）和 Hunt 及 Sahimi（2017）提供了对 EMA 优缺点的全面讨论。Koplik（1981）、Adler 和 Berkowitz（2000）以及其他人（参见 Sahimi 2003）研究了 EMA 准确性的极限。一般来说，EMA（i）如果多孔介质不接近其渗透阈值，即渗透率和电导率消失的临界孔隙率，则准确；（ii）如果它们接近渗透阈值，则对于二维（2D）介质比三维（3D）介质更准确；（iii）对于所谓的临界区域，在三维多孔介质中不太准确。在随机介质中，临界区域大致由 Sahimi（1994）定义，其中 Z 是平均孔隙连通性，而 ${\varphi }_c$ 是临界孔隙率。（iv）如果孔隙大小之间存在扩展相关性，则 EMA 在完全随机多孔介质中的准确性较低，尽管 Mukhopadhyay 和 Sahimi（2000）提出了考虑这种相关性的方法。 与之前提出的理论预测和实验数据的广泛比较与这一图景一致，即如果多孔介质的孔隙率不是极低，以至于几乎不连通，那么本文中开发的 MFA 与 EMA 相结合，可以准确预测多孔介质的有效渗透率。

8.3 Effect of Structural Changes in the Pore Space
8.3 孔隙空间结构变化的影响

In some cases, such as the Cambrian and Fahler sandstones, the effective permeability at high pressures decreases a bit more slowly than the predictions. One possible reason for this is that the morphology of the porous media undergoes fundamental changes at high pressures, such as opening up new cracks that provide new flow paths for the fluid and, hence, arrest to some extent the decline in the permeability at high pressures. If such changes do occur, the approach that we propose in the present paper would not be applicable.
在某些情况下，例如寒武纪和法勒砂岩，高压下的有效渗透率下降速度比预测略慢。一个可能的原因是，在高压下，多孔介质的形态发生根本变化，例如新裂缝的开启为流体提供了新的流动路径，从而在一定程度上减缓了高压下渗透率的下降。如果发生此类变化，本文中提出的方法将不再适用。

8.4 Effect of Microcracks
8.4 微裂缝的影响

If the porous medium contains, in addition to pores, microcracks, then, two approaches may be taken to take their effect into account. One is by simply assuming that the cracks are large pores and use a bimodal PSD to represent both pores and microcracks. This is, of course, a crude approximation whose accuracy remains to be tested.
如果多孔介质除了孔隙外还含有微裂缝，那么可以采取两种方法来考虑它们的影响。一种方法是将裂缝简单地视为大孔隙，并使用双峰 PSD 来表示孔隙和微裂缝。这当然是一种粗略的近似，其准确性还有待验证。

The second method is based on treating the microcracks as completely distinct from the pores such that they may, for example, have their own “network.” In that case an EMA, developed by Hughes and Sahimi (1993a, b) for flow and transport in porous media with two distinct types of flow and transport paths—pores and microcracks—may be used. One would need, of course, not only the PSD, but also the distribution of the cracks’ flow properties. In addition, hydrostatic deformation of a porous medium with cracks typically involves an initial nonlinear elastic crack closure, followed by pore deformation. This implies that one should also develop an expression for the way the microcracks close, since it is different from that for pores that we presented here.
第二种方法是将微裂缝视为与孔隙完全不同的，例如，它们可能有自己的“网络”。在这种情况下，可以使用 Hughes 和 Sahimi（1993a，b）开发的 EMA，该 EMA 用于具有两种不同类型流动和传输路径（孔隙和微裂缝）的多孔介质中的流动和传输。当然，不仅需要 PSD，还需要裂缝流动特性的分布。此外，含有裂缝的多孔介质的静水变形通常涉及初始的非线性弹性裂缝闭合，然后是孔隙变形。这意味着还应该开发一个关于微裂缝闭合的表达式，因为它与我们在这里提出的孔隙闭合不同。

8.5 Effect of Clay on Pore-Pressure Dependence of the Permeability
8.5 黏土对渗透率孔隙压力依赖性的影响

It has been suggested that the permeability of sandstones that are rich in their clay content may be sensitive to pore pressure (Al-Wandy and Zimmerman 2004; Meng et al. 2020). For example, Al-Wandy and Zimmerman (2004) reported that the permeability is more sensitive to changes in the pore pressure than to variations in the confining pressure, and that it increases with increasing pore pressure, but decreases with increasing confining pressure (which is the case in the data that we present and discuss). The model that we have proposed in this paper cannot, in its present form, take this effect into account. If, however, the dependence on the pre pressure of the permeability of a pore and, therefore, the pore’s flow velocity, can be expressed by a functional form, then, the EMA can be extended to include such effects (see, for example, Sahimi 2003). This will be described in a future paper.
有研究表明，富含粘土的砂岩的渗透率可能对孔隙压力敏感（Al-Wandy 和 Zimmerman 2004；Meng 等人 2020 年）。例如，Al-Wandy 和 Zimmerman（2004 年）报告说，渗透率对孔隙压力变化比围压变化更敏感，并且随着孔隙压力的增加而增加，但随围压的增加而降低（这是我们展示和讨论的数据中的情况）。我们在这篇论文中提出的模型在其当前形式下无法考虑这种效应。然而，如果孔隙渗透率及其孔隙流速对预压力的依赖关系可以用函数形式表示，那么 EMA 可以扩展以包括这种效应（例如，参见 Sahimi 2003）。这将在未来的论文中描述。

8.6 Effect of Contact Law for the Grains
8.6 粒子接触定律的影响

The theory that was developed in Section 2 in order to determine the change in the size of a pore as a result of deformation of a porous medium was based on the Hertz–Mindlin theory of contacting grains in unconsolidated porous media. The experimental data that were compared with the theoretical predictions were, however, for mostly consolidated sandstones that have been cemented. It is known (Dvorking and Yin 1995) that cementation influences strongly the contact laws. This could provide an explanation as to why the fitted Young’s moduli of the various sandstones (see above) did not often agree with what one might expect for such porous media, which contain a significant amount of quartz.
该理论是在第 2 节中开发的，旨在确定孔隙大小随多孔介质变形而变化的情况，其基础是未固结多孔介质中接触颗粒的 Hertz-Mindlin 理论。然而，与理论预测进行比较的实验数据主要是已经固结的砂岩。众所周知（Dvorking 和 Yin 1995），胶结强烈影响接触定律。这可以解释为什么各种砂岩的拟合杨氏模量（见上文）并不经常与人们可能期望的这种含有大量石英的孔隙介质相一致。

Thus, one way to address the shortcoming is to use the Hertz–Mindlin theory for cemented sandstones, rederive the expression for the change in the effective size of the pores, and recompute everything. An alternative, and perhaps simpler, approach would be to determine the change in the radius of a hollow cylinder (a pore throat), embedded in a solid material of a given Young’s modulus, as a result of exposing the system to a hydrostatic pressure. This would indeed represent a MFA. The result can then be used to determine the modified PSD and, hence, the effective permeability. We will report on both approaches in a future paper.
因此，解决这一不足的一种方法就是使用水泥砂岩的赫兹-米林理论，重新推导孔隙有效尺寸变化的表达式，并重新计算一切。另一种可能更简单的方法是确定一个空心圆柱（孔隙喉道）的半径变化，该圆柱嵌入具有给定杨氏模量的固体材料中，这是由于将系统暴露在静水压力下。这确实代表了一种 MFA。然后，可以使用这些结果来确定修改后的 PSD，从而确定有效渗透率。我们将在未来的论文中报告这两种方法。

8.7 The Effect of a Grain-Size Distribution
8.7 粒径分布的影响

As described earlier, the present theory of grain deformation, Eqs. (16) and (17), requires an average grain size $R_g$. This is due to the fact that, as emphasized earlier, the present theory of deformation is a MFA and, as such, it considers the deformation of only two neighboring grains and the change in the effective radius of the pore between them. Thus, if a grain-size distribution (GSD) is available (see, for example, Cheung et al. 2012), one can compute $R_g$. Beyond that, and in order to take into account the effect of a GSD of a collection of grains, the MFA must be refined, and the effect of the interactions between a collection of grains must be taken into account. While numerical simulations in this direction have been made in the past (see, for example, Bakhshian and Sahimi 2016; Das and Singh 2017), to our knowledge none has produced a tractable theory for the evolution of the PSD. This is an issue that we are currently studying.
如前所述，当前关于颗粒变形的理论，即公式（16）和（17），需要平均颗粒尺寸 $R_g$ 。这是因为，如前所述，当前变形理论是 MFA 理论，因此它只考虑了两个相邻颗粒的变形以及它们之间孔隙有效半径的变化。因此，如果有一个颗粒尺寸分布（GSD）（例如，参见 Cheung 等人 2012 年的研究），就可以计算出 $R_g$ 。除此之外，为了考虑颗粒集合的 GSD 的影响，MFA 必须得到改进，并且必须考虑颗粒集合之间的相互作用。虽然过去已经在这方面进行了数值模拟（例如，参见 Bakhshian 和 Sahimi 2016 年；Das 和 Singh 2017 年的研究），但据我们所知，还没有产生一个关于 PSD 演化的可处理理论。这是我们目前正在研究的问题。

8.8 Effect of the Deformation Mode
8.8 变形模式的影响

Deformation of porous geological formations, such as depleted oil reservoirs, is often caused by uniaxial stress. What we have studied is the case in which the confining pressure is applied hydrostatically. It is clear that the deformations that result from the two types of the boundary conditions are different, because the spatial distributions of pressure in the two systems are different. But, when, for example, the overburden pressure exerts itself uniaxially in an oil reservoir, the surrounding rock limits the resulting lateral deformation. This implies that one obtains mostly vertical compaction, which represents smaller changes in the pore sizes than what is caused by hydrostatic pressure. Section 7 demonstrated that our theory provides accurate predictions for the macroscopic permeability as a function of the hydrostatic pressure, and such a pressure deforms the pore space much more extensively than a uniaxial stress would. We therefore believe that a slightly modified theory would be at least equally accurate for the case in which a uniaxial stress is exerted on a porous medium.
多孔地质构造，如枯竭的油田，的变形通常是由单轴应力引起的。我们所研究的是施加静水压力的案例。很明显，由两种边界条件引起的变形是不同的，因为两个系统中的压力空间分布不同。但是，例如，当覆盖压力在油藏中单轴施加时，周围岩石限制了由此产生的横向变形。这意味着主要获得垂直压缩，这比静水压力引起的孔隙尺寸变化要小。第 7 节证明了我们的理论可以准确预测宏观渗透率作为静水压力的函数，而这种压力比单轴应力对孔隙空间的变形要广泛得多。因此，我们认为对理论进行轻微修改，对于施加单轴应力在多孔介质上的情况，至少同样准确。

8.9 Universal Curves for Rescaled Permeability versus Rescaled Pressure
8.9 重标渗透率与重标压力的通用曲线

We have observed that, for at least some of the sandstones that we describe earlier, if we consider the $K_e(P)/K_0$ curves and rescale the pressure to a suitably selected rescaled form, we obtain a single, more or less universal curve, often referred to as the master curve. Physically, the master curve represents essentially the dimensionless response of a porous medium to the changes in the pressure, since the initial PSD and the Poisson’s ratio are all set to constant values. One clue to the proper rescaling of P is provided by Eq. (17), since it implies that, with $R\approx R_g$, the quantity $u/R_g$ is a function only of $P/E_e$.
我们观察到，对于我们所描述的至少一些砂岩，如果我们考虑 $K_e(P)/K_0$ 曲线并将压力重新缩放到一个合适选择的缩放形式，我们得到一条单一、相对通用的曲线，通常被称为主曲线。从物理上讲，主曲线基本上代表了多孔介质对压力变化的无量纲响应，因为初始 PSD 和泊松比都被设定为常数。P 正确缩放的线索之一由公式(17)提供，因为它暗示了，在 $R\approx R_g$ 的情况下，量 $u/R_g$ 仅是 $P/E_e$ 的函数。

The existence of such a master curve implies that it can predict the measurements by rescaling the pressure axis to generate a dimensionless form based on the input data—the initial porosity and an experimental data point that provides an estimate for the effective Young’s modulus $E_e$. Other mechanical models than the HM theory of point-contact that we utilized in this paper may provide a different master curve (based on different rescaled variables). But, the general implication is clear: with proper rescaling of the main variables, one may obtain a universal curve that provides accurate predictions for a variety of deforming porous media. In fact, such a master curve was proposed much earlier (Rassamdana et al. 1996) for another property of oil reservoirs that represent large-scale porous media.
这样一条主曲线的存在意味着它可以通过对压力轴进行缩放来预测测量值，生成基于输入数据（初始孔隙率和提供有效杨氏模量估计的实验数据点）的无量纲形式——0# $E_e$ 。除了本文中使用的点接触 HM 理论之外的其他力学模型可能提供不同的主曲线（基于不同的缩放变量）。但，一般含义是明确的：通过适当缩放主要变量，可以获得一个适用于各种变形多孔介质的通用曲线，提供准确的预测。事实上，这样的主曲线早在（Rassamdana 等人，1996 年）就被提出，用于表示大型多孔介质的油藏的另一性质。

9 Summary
9 摘要

Predicting physical properties of deforming porous media, and in particular their permeability and transport characteristics, such as their electrical conductivity when they are saturated with brine, is important to many physical processes. They include shale formations undergoing fracking, and oil, gas, and coal-bed reservoirs, as well as composite materials that are used in everyday life. We presented a new theoretical model for predicting the permeability $K_e$ of such porous media and materials. The theory, a mean-field approximation, determines the change in the size of a pore between two grains that deform when a hydrostatic pressure is applied to them. Then, given the initial PSD of a porous medium before deformation and the Young’s modulus of the grains as the input, the theory determines the PSD of a porous medium that is deformed by applying an external pressure P, which is then used with the effective-medium approximation to predict the effective permeability of the porous medium at the same pressure. Extensive comparison between the theoretical predictions and experimental data for the pressure-dependence of the effective permeabilities of twenty four sandstones indicated agreement between the two in almost all cases, ranging from very good to excellent.
预测变形多孔介质的物理性质，特别是它们的渗透性和传输特性，如它们在饱和盐水时的电导率，对于许多物理过程至关重要。这包括正在经历压裂的页岩层、油气和煤层储层，以及日常生活中使用的复合材料。我们提出了一种新的理论模型来预测此类多孔介质和材料的渗透率。该理论，一种平均场近似，确定了在施加静水压力时，两个变形颗粒之间孔隙大小的变化。然后，给定变形前多孔介质的初始 PSD 和颗粒的杨氏模量作为输入，该理论确定了在外部压力 P 作用下变形的多孔介质的 PSD，然后使用有效介质近似来预测在相同压力下多孔介质的有效渗透率。 对二十四种砂岩的有效渗透率压力依赖性的理论预测与实验数据的广泛比较表明，在几乎所有情况下，两者之间都表现出良好至极好的吻合。

With some modifications, the same approach can be used for predicting the electrical conductivity of brine-saturated porous media that undergo deformation, as well as their relative permeabilities to two-phase flows. Work in this direction is in progress. The results will be reported in Part II of this series (Richesson and Sahimi 2021).
通过一些修改，相同的方法可以用于预测受变形的盐水饱和多孔介质的电导率以及它们对两相流的相对渗透率。这方面的研究正在进行中。结果将在本系列的第二部分中报告（Richesson 和 Sahimi 2021）。